The ideal attenuator with (power) attenuation ![]() is frequency
independent and the model is valid for DC and for AC simulation.
It is determined by the following Z parameters.
is frequency
independent and the model is valid for DC and for AC simulation.
It is determined by the following Z parameters.
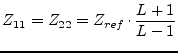 |
(9.72) |
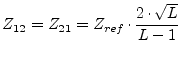 |
(9.73) |
The Z parameter representation is not very practical as new lines in the MNA matrix have to be added. More useful are the Y parameters.
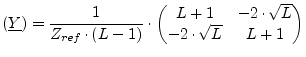 |
(9.74) |
Attenuator with (power) attenuation ![]() , reference impedance
, reference impedance ![]() and temperature
and temperature ![]() :
:
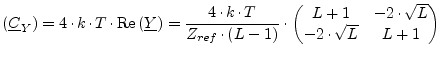 |
(9.75) |
The scattering parameters and noise wave correlation matrix of an
ideal attenuator with (power) attenuation ![]() (loss) (or power gain
(loss) (or power gain
![]() ) in reference to the impedance
) in reference to the impedance ![]() writes as follows.
writes as follows.
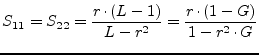 |
(9.76) |
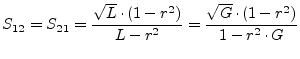 |
(9.77) |
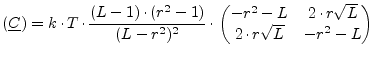 |
(9.78) |
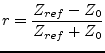 |
(9.79) |