Namespaces | |
| namespace | detail |
| namespace | device |
| namespace | emi |
| namespace | eqn |
| namespace | fourier |
| namespace | transient |
Data Structures | |
| class | acsolver |
| class | analysis |
| class for performing circuit analyses. More... | |
Functions | |
| static void | euclidian_update (nr_double_t a, nr_double_t &n, nr_double_t &scale) |
| template<typename nr_type_t > | |
| nr_type_t | cond_conj (nr_type_t t) |
| template<> | |
| double | cond_conj (double t) |
| static nr_double_t | givens (nr_double_t a, nr_double_t b, nr_double_t &c, nr_double_t &s) |
| Helper function computes Givens rotation. | |
| static char * | Cplx2String (nr_complex_t c) |
| nr_complex_t | cos (const nr_complex_t z) |
| Compute complex cosine. | |
| nr_complex_t | sin (const nr_complex_t z) |
| Compute complex sine. | |
| nr_complex_t | tan (const nr_complex_t z) |
| Compute complex tangent. | |
| nr_complex_t | acos (const nr_complex_t z) |
| Compute complex arc cosine. | |
| nr_complex_t | asin (const nr_complex_t z) |
| Compute complex arc sine. | |
| nr_complex_t | atan (const nr_complex_t z) |
| Compute complex arc tangent. | |
| nr_complex_t | cosh (const nr_complex_t z) |
| Compute complex hyperbolic cosine. | |
| nr_complex_t | sinh (const nr_complex_t z) |
| Compute complex hyperbolic sine. | |
| nr_complex_t | tanh (const nr_complex_t z) |
| Compute complex hyperbolic tangent. | |
| nr_complex_t | acosh (const nr_complex_t z) |
| Compute complex arc hyperbolic cosine. | |
| nr_complex_t | asinh (const nr_complex_t z) |
| Compute complex arc hyperbolic sine. | |
| nr_complex_t | atanh (const nr_complex_t z) |
| Compute complex arc hyperbolic tangent. | |
| nr_complex_t | exp (const nr_complex_t z) |
| Compute complex exponential. | |
| nr_complex_t | log (const nr_complex_t z) |
| Compute principal value of natural logarithm of z. | |
| nr_complex_t | log10 (const nr_complex_t z) |
| Compute principal value of decimal logarithm of z. | |
| nr_complex_t | pow (const nr_complex_t z, const nr_double_t d) |
| Compute power function with real exponent. | |
| nr_complex_t | pow (const nr_double_t d, const nr_complex_t z) |
| Compute power function with complex exponent but real mantisse. | |
| nr_complex_t | pow (const nr_complex_t z1, const nr_complex_t z2) |
| Compute complex power function. | |
| nr_complex_t | sqrt (const nr_complex_t z) |
| Compute principal value of square root. | |
| nr_double_t | norm (const nr_complex_t z) |
| Compute euclidian norm of complex number. | |
| nr_complex_t | cot (const nr_complex_t z) |
| Compute complex cotangent. | |
| nr_complex_t | acot (const nr_complex_t z) |
| Compute complex arc cotangent. | |
| nr_complex_t | coth (const nr_complex_t z) |
| Compute complex hyperbolic cotangent. | |
| nr_complex_t | acoth (const nr_complex_t z) |
| Compute complex argument hyperbolic cotangent. | |
| nr_complex_t | sech (const nr_complex_t z) |
| Compute complex hyperbolic secant. | |
| nr_complex_t | asech (const nr_complex_t z) |
| Compute complex argument hyperbolic secant. | |
| nr_complex_t | cosech (const nr_complex_t z) |
| Compute complex argument hyperbolic cosec. | |
| nr_complex_t | atan2 (const nr_complex_t y, const nr_complex_t x) |
| Compute complex arc tangent fortran like function. | |
| nr_complex_t | log2 (const nr_complex_t z) |
| Compute principal value of binary logarithm of z. | |
| nr_complex_t | signum (const nr_complex_t z) |
| complex signum function | |
| nr_complex_t | sign (const nr_complex_t z) |
| complex sign function | |
| nr_complex_t | sinc (const nr_complex_t z) |
| Cardinal sine. | |
| nr_double_t | xhypot (const nr_complex_t a, const nr_complex_t b) |
| Euclidean distance function for complex argument. | |
| nr_double_t | xhypot (nr_double_t a, nr_complex_t b) |
| Euclidean distance function for a double b complex. | |
| nr_double_t | xhypot (nr_complex_t a, nr_double_t b) |
| Euclidean distance function for b double a complex. | |
| nr_complex_t | round (const nr_complex_t z) |
| Complex round Round is the nearest integral value Apply round to real and imaginary part. | |
| nr_complex_t | trunc (const nr_complex_t z) |
| Complex trunc Apply round to integer, towards zero to real and imaginary part. | |
| nr_double_t | dB (const nr_complex_t z) |
Magnitude in dB Compute 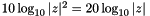 . . | |
| nr_complex_t | limexp (const nr_complex_t z) |
| Compute limited complex exponential. | |
| nr_complex_t | polar (const nr_double_t mag, const nr_double_t ang) |
| Construct a complex number using polar notation. | |
| nr_complex_t | polar (const nr_complex_t a, const nr_complex_t p) |
| Extension of polar construction to complex. | |
| nr_complex_t | ztor (const nr_complex_t z, nr_complex_t zref) |
| Converts impedance to reflexion coefficient. | |
| nr_complex_t | rtoz (const nr_complex_t r, nr_complex_t zref) |
| Converts reflexion coefficient to impedance. | |
| nr_complex_t | ytor (const nr_complex_t y, nr_complex_t zref) |
| Converts admittance to reflexion coefficient. | |
| nr_complex_t | rtoy (const nr_complex_t r, nr_complex_t zref) |
| Converts reflexion coefficient to admittance. | |
| nr_complex_t | floor (const nr_complex_t z) |
| Complex floor. | |
| nr_complex_t | ceil (const nr_complex_t z) |
| Complex ceil Ceil is the smallest integral value not less than argument Apply ceil to real and imaginary part. | |
| nr_complex_t | fix (const nr_complex_t z) |
| Complex fix. | |
| nr_complex_t | fmod (const nr_complex_t x, const nr_complex_t y) |
| Complex fmod Apply fmod to the complex z. | |
| nr_complex_t | sqr (const nr_complex_t z) |
| Square of complex number. | |
| nr_complex_t | step (const nr_complex_t z) |
| Heaviside step function for complex number. | |
| nr_complex_t | cbesselj (unsigned int n, nr_complex_t z) |
| Main entry point for besselj function. | |
| static nr_complex_t | cbesselj_smallarg (unsigned int n, nr_complex_t z) |
| static nr_complex_t | cbesselj_mediumarg_odd (unsigned int n, nr_complex_t z) |
| static nr_complex_t | cbesselj_mediumarg_even (unsigned int n, nr_complex_t z) |
| static nr_complex_t | cbesselj_mediumarg (unsigned int n, nr_complex_t z) |
| static nr_complex_t | cbesselj_largearg (unsigned int n, nr_complex_t z) |
| besselj for large argument | |
| nr_complex_t | jn (const int n, const nr_complex_t z) |
| Bessel function of first kind. | |
| nr_complex_t | yn (const int n, const nr_complex_t z) |
| Bessel function of second kind. | |
| nr_complex_t | i0 (const nr_complex_t z) |
| Modified Bessel function of first kind. | |
| nr_complex_t | erf (const nr_complex_t z) |
| Error function. | |
| nr_complex_t | erfc (const nr_complex_t z) |
| Complementart error function. | |
| nr_complex_t | erfinv (const nr_complex_t z) |
| Inverse of error function. | |
| nr_complex_t | erfcinv (const nr_complex_t z) |
| Inverse of complementart error function. | |
| nr_complex_t | operator% (const nr_complex_t z1, const nr_complex_t z2) |
| Modulo. | |
| nr_complex_t | operator% (const nr_complex_t z1, const nr_double_t r2) |
| Modulo. | |
| nr_complex_t | operator% (const nr_double_t r1, const nr_complex_t z2) |
| Modulo. | |
| bool | operator== (const nr_complex_t z1, const nr_complex_t z2) |
| Equality of two complex. | |
| bool | operator!= (const nr_complex_t z1, const nr_complex_t z2) |
| Inequality of two complex. | |
| bool | operator>= (const nr_complex_t z1, const nr_complex_t z2) |
| Superior of equal. | |
| bool | operator<= (const nr_complex_t z1, const nr_complex_t z2) |
| Inferior of equal. | |
| bool | operator> (const nr_complex_t z1, const nr_complex_t z2) |
| Superior. | |
| bool | operator< (const nr_complex_t z1, const nr_complex_t z2) |
| Inferior. | |
| nr_double_t | rad2deg (const nr_complex_t x) |
| rad2deg Convert radian to degree | |
| nr_double_t | deg2rad (const nr_complex_t x) |
| rad2deg Convert radian to degree | |
| matrix | operator+ (matrix a, matrix b) |
| Matrix addition. | |
| matrix | operator- (matrix a, matrix b) |
| Matrix subtraction. | |
| matrix | operator* (matrix a, nr_complex_t z) |
| Matrix scaling complex version. | |
| matrix | operator* (nr_complex_t z, matrix a) |
| Matrix scaling complex version (different order) | |
| matrix | operator* (matrix a, nr_double_t d) |
| Matrix scaling complex version. | |
| matrix | operator* (nr_double_t d, matrix a) |
| Matrix scaling real version (different order) | |
| matrix | operator/ (matrix a, nr_complex_t z) |
| Matrix scaling division by complex version. | |
| matrix | operator/ (matrix a, nr_double_t d) |
| Matrix scaling division by real version. | |
| matrix | operator* (matrix a, matrix b) |
| matrix | operator+ (matrix a, nr_complex_t z) |
| Complex scalar addition. | |
| matrix | operator+ (nr_complex_t z, matrix a) |
| Complex scalar addition different order. | |
| matrix | operator+ (matrix a, nr_double_t d) |
| Real scalar addition. | |
| matrix | operator+ (nr_double_t d, matrix a) |
| Real scalar addition different order. | |
| matrix | operator- (matrix a, nr_complex_t z) |
| Complex scalar substraction. | |
| matrix | operator- (nr_complex_t z, matrix a) |
| Complex scalar substraction different order. | |
| matrix | operator- (matrix a, nr_double_t d) |
| Real scalar substraction. | |
| matrix | operator- (nr_double_t d, matrix a) |
| Real scalar substraction different order. | |
| matrix | transpose (matrix a) |
| Matrix transposition. | |
| matrix | conj (matrix a) |
| Conjugate complex matrix. | |
| matrix | adjoint (matrix a) |
| adjoint matrix | |
| matrix | abs (matrix a) |
| Computes magnitude of each matrix element. | |
| matrix | dB (matrix a) |
| Computes magnitude in dB of each matrix element. | |
| matrix | arg (matrix a) |
| Computes the argument of each matrix element. | |
| matrix | real (matrix a) |
| Real part matrix. | |
| matrix | imag (matrix a) |
| Imaginary part matrix. | |
| matrix | sqr (matrix a) |
| Multiply a matrix by itself. | |
| matrix | eye (int rs, int cs) |
| Create identity matrix with specified number of rows and columns. | |
| matrix | eye (int s) |
| Create a square identity matrix. | |
| matrix | diagonal (qucs::vector diag) |
| Create a diagonal matrix from a vector. | |
| matrix | pow (matrix a, int n) |
| nr_complex_t | cofactor (matrix a, int u, int v) |
| Computes the complex cofactor of the given determinant. | |
| nr_complex_t | detLaplace (matrix a) |
| Compute determinant of the given matrix using Laplace expansion. | |
| nr_complex_t | detGauss (matrix a) |
| Compute determinant Gaussian algorithm. | |
| nr_complex_t | det (matrix a) |
| Compute determinant of the given matrix. | |
| matrix | inverseLaplace (matrix a) |
| Compute inverse matrix using Laplace expansion. | |
| matrix | inverseGaussJordan (matrix a) |
| Compute inverse matrix using Gauss-Jordan elimination. | |
| matrix | inverse (matrix a) |
| Compute inverse matrix. | |
| matrix | stos (matrix s, qucs::vector zref, qucs::vector z0) |
| S params to S params. | |
| matrix | stos (matrix s, nr_complex_t zref, nr_complex_t z0) |
| S renormalization with all part identic. | |
| matrix | stos (matrix s, nr_double_t zref, nr_double_t z0) |
| S renormalization with all part identic and real. | |
| matrix | stos (matrix s, qucs::vector zref, nr_complex_t z0) |
| S renormalization (variation) | |
| matrix | stos (matrix s, nr_complex_t zref, qucs::vector z0) |
| S renormalization (variation) | |
| matrix | stoz (matrix s, qucs::vector z0) |
| Scattering parameters to impedance matrix. | |
| matrix | stoz (matrix s, nr_complex_t z0) |
| Scattering parameters to impedance matrix identic case. | |
| matrix | ztos (matrix z, qucs::vector z0) |
| Convert impedance matrix scattering parameters. | |
| matrix | ztos (matrix z, nr_complex_t z0) |
| Convert impedance matrix to scattering parameters identic case. | |
| matrix | ztoy (matrix z) |
| impedance matrix to admittance matrix. | |
| matrix | stoy (matrix s, qucs::vector z0) |
| Scattering parameters to admittance matrix. | |
| matrix | stoy (matrix s, nr_complex_t z0) |
| Convert scattering pto adminttance parameters identic case. | |
| matrix | ytos (matrix y, qucs::vector z0) |
| Admittance matrix to scattering parameters. | |
| matrix | ytos (matrix y, nr_complex_t z0) |
| Convert Admittance matrix to scattering parameters identic case. | |
| matrix | stoa (matrix s, nr_complex_t z1, nr_complex_t z2) |
| Converts chain matrix to scattering parameters. | |
| matrix | atos (matrix a, nr_complex_t z1, nr_complex_t z2) |
| Converts chain matrix to scattering parameters. | |
| matrix | stoh (matrix s, nr_complex_t z1, nr_complex_t z2) |
| Converts scattering parameters to hybrid matrix. | |
| matrix | htos (matrix h, nr_complex_t z1, nr_complex_t z2) |
| Converts hybrid matrix to scattering parameters. | |
| matrix | stog (matrix s, nr_complex_t z1, nr_complex_t z2) |
| matrix | gtos (matrix g, nr_complex_t z1, nr_complex_t z2) |
| matrix | ytoz (matrix y) |
| Convert admittance matrix to impedance matrix. | |
| matrix | cytocs (matrix cy, matrix s) |
| Admittance noise correlation matrix to S-parameter noise correlation matrix. | |
| matrix | cstocy (matrix cs, matrix y) |
| Converts S-parameter noise correlation matrix to admittance noise correlation matrix. | |
| matrix | cztocs (matrix cz, matrix s) |
| Converts impedance noise correlation matrix to S-parameter noise correlation matrix. | |
| matrix | cstocz (matrix cs, matrix z) |
| Converts S-parameter noise correlation matrix to impedance noise correlation matrix. | |
| matrix | cztocy (matrix cz, matrix y) |
| Converts impedance noise correlation matrix to admittance noise correlation matrix. | |
| matrix | cytocz (matrix cy, matrix z) |
| Converts admittance noise correlation matrix to impedance noise correlation matrix. | |
| matrix | twoport (matrix m, char in, char out) |
| Generic conversion matrix. | |
| matrix | diagonal (vector) |
| matrix | stos (matrix, vector, nr_complex_t z0=50.0) |
| matrix | stos (matrix, nr_complex_t, vector) |
| matrix | stos (matrix, vector, vector) |
| matrix | stoz (matrix, vector) |
| matrix | ztos (matrix, vector) |
| matrix | stoy (matrix, vector) |
| matrix | ytos (matrix, vector) |
| nr_double_t | rollet (matrix) |
| nr_double_t | b1 (matrix) |
| matrix | rad2deg (matrix) |
| matrix | deg2rad (matrix) |
| nr_double_t | cos (const nr_double_t arg) |
| Compute cosine of an angle. | |
| nr_double_t | sin (const nr_double_t arg) |
| Compute sine of an angle. | |
| nr_double_t | tan (const nr_double_t arg) |
| Compute tangent of an angle. | |
| nr_double_t | acos (const nr_double_t arg) |
| Compute arc cosine. | |
| nr_double_t | asin (const nr_double_t arg) |
| Compute arc sine. | |
| nr_double_t | atan (const nr_double_t arg) |
| Compute arc tangent. | |
| nr_double_t | atan2 (const nr_double_t x, const nr_double_t y) |
| Compute arc tangent with two parameters (fortran like function) | |
| nr_double_t | cosh (const nr_double_t arg) |
| Compute hyperbolic cosine. | |
| nr_double_t | sinh (const nr_double_t arg) |
| Compute hyperbolic sine. | |
| nr_double_t | tanh (const nr_double_t arg) |
| Compute hyperbolic tangent. | |
| nr_double_t | acosh (const nr_double_t arg) |
| Compute arc hyperbolic cosine. | |
| nr_double_t | asinh (const nr_double_t arg) |
| Compute arc hyperbolic sine. | |
| nr_double_t | atanh (const nr_double_t arg) |
| Compute arc hyperbolic tangent. | |
| nr_double_t | exp (const nr_double_t arg) |
| nr_double_t | log (const nr_double_t arg) |
| nr_double_t | log10 (const nr_double_t arg) |
| nr_double_t | pow (const nr_double_t a, const nr_double_t b) |
| nr_double_t | sqrt (const nr_double_t d) |
| nr_double_t | xhypot (const nr_double_t a, const nr_double_t b) |
| Euclidean distance function. | |
| nr_double_t | erf (nr_double_t arg) |
| nr_double_t | ceil (nr_double_t arg) |
| nr_double_t | floor (nr_double_t arg) |
| nr_double_t | fmod (nr_double_t arg) |
| nr_double_t | trunc (nr_double_t arg) |
| nr_double_t | round (nr_double_t arg) |
| nr_double_t | coth (const nr_double_t d) |
| nr_double_t | sech (const nr_double_t d) |
| nr_double_t | cosech (const nr_double_t d) |
| nr_double_t | sqr (const nr_double_t r) |
| Square a value. | |
| unsigned int | sqr (unsigned int r) |
| nr_double_t | quadr (const nr_double_t r) |
| Quartic function. | |
| nr_double_t | limexp (const nr_double_t r) |
| Compute limited exponential. | |
| nr_double_t | signum (const nr_double_t d) |
| real signum function | |
| nr_double_t | sign (const nr_double_t d) |
| real sign function | |
| nr_double_t | sinc (const nr_double_t d) |
| Real cardinal sinus. | |
| nr_double_t | fix (const nr_double_t d) |
| Fix function. | |
| nr_double_t | step (const nr_double_t d) |
| Heaviside step function. | |
| unsigned int | factorial (unsigned int n) |
| Compute factorial n ie $n!$. | |
| nr_double_t | real (const nr_double_t r) |
| Real part of real number. | |
| nr_double_t | imag (const nr_double_t r) |
| Imaginary part of complex number. | |
| nr_double_t | norm (const nr_double_t r) |
| Compute euclidian norm of real number. | |
| nr_double_t | abs (const nr_double_t r) |
| Compute complex modulus of real number. | |
| nr_double_t | conj (const nr_double_t r) |
| Conjugate of real number. | |
| nr_double_t | rad2deg (const nr_double_t x) |
| rad2deg Convert radian to degree | |
| nr_double_t | deg2rad (const nr_double_t x) |
| deg2rad Convert radian to degree | |
| static nr_double_t | cubic (const nr_double_t x) |
| Compute the third power of input. | |
| static nr_double_t | celsius2kelvin (const nr_double_t x) |
| Convert Celsius to Kelvin. | |
| static nr_double_t | kelvin2celsius (const nr_double_t x) |
| Convert Kelvin to Celsius. | |
| matvec | operator+ (matvec a, matvec b) |
| matvec | operator+ (matvec a, matrix b) |
| matvec | operator+ (matvec a, qucs::vector b) |
| matvec | operator+ (qucs::vector b, matvec a) |
| matvec | operator+ (matrix a, matvec b) |
| matvec | operator+ (matvec a, nr_complex_t z) |
| matvec | operator+ (nr_complex_t z, matvec a) |
| matvec | operator+ (matvec a, nr_double_t d) |
| matvec | operator+ (nr_double_t d, matvec a) |
| matvec | operator- (matvec a, nr_complex_t z) |
| matvec | operator- (nr_complex_t z, matvec a) |
| matvec | operator- (matvec a, nr_double_t d) |
| matvec | operator- (nr_double_t d, matvec a) |
| matvec | operator- (matvec a, matvec b) |
| matvec | operator- (matvec a, matrix b) |
| matvec | operator- (matrix a, matvec b) |
| matvec | operator- (matvec a, qucs::vector b) |
| matvec | operator- (qucs::vector b, matvec a) |
| matvec | operator* (matvec a, nr_complex_t z) |
| matvec | operator* (nr_complex_t z, matvec a) |
| matvec | operator* (matvec a, nr_double_t d) |
| matvec | operator* (nr_double_t d, matvec a) |
| matvec | operator* (matvec a, qucs::vector b) |
| matvec | operator* (qucs::vector a, matvec b) |
| matvec | operator/ (matvec a, nr_complex_t z) |
| matvec | operator/ (matvec a, nr_double_t d) |
| matvec | operator/ (matvec a, qucs::vector b) |
| matvec | operator* (matvec a, matvec b) |
| matvec | operator* (matvec a, matrix b) |
| matvec | operator* (matrix a, matvec b) |
| qucs::vector | det (matvec a) |
| matvec | inverse (matvec a) |
| matvec | sqr (matvec a) |
| matvec | pow (matvec a, int n) |
| matvec | pow (matvec a, qucs::vector v) |
| matvec | conj (matvec a) |
| matvec | abs (matvec a) |
| matvec | dB (matvec a) |
| matvec | arg (matvec a) |
| matvec | real (matvec a) |
| matvec | imag (matvec a) |
| matvec | adjoint (matvec a) |
| matvec | transpose (matvec a) |
| matvec | stos (matvec s, qucs::vector zref, qucs::vector z0) |
| matvec | stos (matvec s, nr_complex_t zref, nr_complex_t z0) |
| matvec | stos (matvec s, nr_double_t zref, nr_double_t z0) |
| matvec | stos (matvec s, qucs::vector zref, nr_complex_t z0) |
| matvec | stos (matvec s, nr_complex_t zref, qucs::vector z0) |
| matvec | stoy (matvec s, qucs::vector z0) |
| matvec | stoy (matvec s, nr_complex_t z0) |
| matvec | ytos (matvec y, qucs::vector z0) |
| matvec | ytos (matvec y, nr_complex_t z0) |
| matvec | stoz (matvec s, qucs::vector z0) |
| matvec | stoz (matvec s, nr_complex_t z0) |
| matvec | ztos (matvec z, qucs::vector z0) |
| matvec | ztos (matvec z, nr_complex_t z0) |
| matvec | ztoy (matvec z) |
| matvec | ytoz (matvec y) |
| matvec | twoport (matvec m, char in, char out) |
| qucs::vector | rollet (matvec m) |
| qucs::vector | b1 (matvec m) |
| matvec | rad2deg (matvec a) |
| matvec | deg2rad (matvec a) |
| matvec | pow (matvec, vector) |
| matvec | stos (matvec, vector, nr_complex_t z0=50.0) |
| matvec | stos (matvec, nr_complex_t, vector) |
| matvec | stos (matvec, vector, vector) |
| matvec | stoz (matvec, vector) |
| matvec | ztos (matvec, vector) |
| matvec | stoy (matvec, vector) |
| matvec | ytos (matvec, vector) |
| static int | sortfunc (struct nodelist_t *n) |
| static int | insfunc (struct nodelist_t *n1, struct nodelist_t *n2) |
| template<class nr_type_t > | |
| tmatrix< nr_type_t > | inverse (tmatrix< nr_type_t > a) |
| template<class nr_type_t > | |
| tmatrix< nr_type_t > | teye (int n) |
| template<class nr_type_t > | |
| tmatrix< nr_type_t > | operator* (tmatrix< nr_type_t > a, tmatrix< nr_type_t > b) |
| template<class nr_type_t > | |
| tvector< nr_type_t > | operator* (tmatrix< nr_type_t > a, tvector< nr_type_t > b) |
| template<class nr_type_t > | |
| tvector< nr_type_t > | operator* (tvector< nr_type_t > a, tmatrix< nr_type_t > b) |
| template<class nr_type_t > | |
| tvector< nr_type_t > | operator+ (tvector< nr_type_t > a, tvector< nr_type_t > b) |
| template<class nr_type_t > | |
| tvector< nr_type_t > | operator- (tvector< nr_type_t > a, tvector< nr_type_t > b) |
| template<class nr_type_t > | |
| tvector< nr_type_t > | operator* (nr_double_t s, tvector< nr_type_t > a) |
| template<class nr_type_t > | |
| tvector< nr_type_t > | operator* (tvector< nr_type_t > a, nr_double_t s) |
| template<class nr_type_t > | |
| tvector< nr_type_t > | operator* (tvector< nr_type_t > a, tvector< nr_type_t > b) |
| template<class nr_type_t > | |
| nr_type_t | scalar (tvector< nr_type_t > a, tvector< nr_type_t > b) |
| template<class nr_type_t > | |
| nr_type_t | sum (tvector< nr_type_t > a) |
| template<class nr_type_t > | |
| tvector< nr_type_t > | operator- (tvector< nr_type_t > a) |
| template<class nr_type_t > | |
| bool | operator< (tvector< nr_type_t > a, tvector< nr_type_t > b) |
| template<class nr_type_t > | |
| bool | operator> (tvector< nr_type_t > a, tvector< nr_type_t > b) |
| template<class nr_type_t > | |
| tvector< nr_type_t > | operator+ (nr_type_t s, tvector< nr_type_t > a) |
| template<class nr_type_t > | |
| tvector< nr_type_t > | operator+ (tvector< nr_type_t > a, nr_type_t s) |
| template<class nr_type_t > | |
| nr_double_t | norm (tvector< nr_type_t > a) |
| template<class nr_type_t > | |
| nr_double_t | maxnorm (tvector< nr_type_t > a) |
| template<class nr_type_t > | |
| tvector< nr_type_t > | conj (tvector< nr_type_t > a) |
| vector | unwrap (vector v, nr_double_t tol, nr_double_t step) |
| nr_complex_t | sum (vector v) |
| nr_complex_t | prod (vector v) |
| nr_complex_t | avg (vector v) |
| vector | signum (vector v) |
| vector | sign (vector v) |
| vector | xhypot (vector v, const nr_complex_t z) |
| vector | xhypot (vector v, const nr_double_t d) |
| vector | xhypot (const nr_complex_t z, vector v) |
| vector | xhypot (const nr_double_t d, vector v) |
| vector | xhypot (vector v1, vector v2) |
| vector | sinc (vector v) |
| vector | abs (vector v) |
| vector | norm (vector v) |
| vector | arg (vector v) |
| vector | real (vector v) |
| vector | imag (vector v) |
| vector | conj (vector v) |
| vector | dB (vector v) |
| vector | sqrt (vector v) |
| vector | exp (vector v) |
| vector | limexp (vector v) |
| vector | log (vector v) |
| vector | log10 (vector v) |
| vector | log2 (vector v) |
| vector | pow (vector v, const nr_complex_t z) |
| vector | pow (vector v, const nr_double_t d) |
| vector | pow (const nr_complex_t z, vector v) |
| vector | pow (const nr_double_t d, vector v) |
| vector | pow (vector v1, vector v2) |
| vector | sin (vector v) |
| vector | asin (vector v) |
| vector | acos (vector v) |
| vector | cos (vector v) |
| vector | tan (vector v) |
| vector | atan (vector v) |
| vector | cot (vector v) |
| vector | acot (vector v) |
| vector | sinh (vector v) |
| vector | asinh (vector v) |
| vector | cosh (vector v) |
| vector | sech (vector v) |
| vector | cosech (vector v) |
| vector | acosh (vector v) |
| vector | asech (vector v) |
| vector | tanh (vector v) |
| vector | atanh (vector v) |
| vector | coth (vector v) |
| vector | acoth (vector v) |
| vector | ztor (vector v, nr_complex_t zref) |
| vector | ytor (vector v, nr_complex_t zref) |
| vector | rtoz (vector v, nr_complex_t zref) |
| vector | rtoy (vector v, nr_complex_t zref) |
| vector | diff (vector var, vector dep, int n) |
| vector | operator+ (vector v1, vector v2) |
| vector | operator+ (vector v, const nr_complex_t c) |
| vector | operator+ (const nr_complex_t c, vector v) |
| vector | operator+ (vector v, const nr_double_t d) |
| vector | operator+ (const nr_double_t d, vector v) |
| vector | operator- (vector v1, vector v2) |
| vector | operator- (vector v, const nr_complex_t c) |
| vector | operator- (vector v, const nr_double_t d) |
| vector | operator- (const nr_complex_t c, vector v) |
| vector | operator- (const nr_double_t d, vector v) |
| vector | operator* (vector v1, vector v2) |
| vector | operator* (vector v, const nr_complex_t c) |
| vector | operator* (vector v, const nr_double_t d) |
| vector | operator* (const nr_complex_t c, vector v) |
| vector | operator* (const nr_double_t d, vector v) |
| vector | operator/ (vector v1, vector v2) |
| vector | operator/ (vector v, const nr_complex_t c) |
| vector | operator/ (vector v, const nr_double_t d) |
| vector | operator/ (const nr_complex_t c, vector v) |
| vector | operator/ (const nr_double_t d, vector v) |
| vector | operator% (vector v, const nr_complex_t z) |
| vector | operator% (vector v, const nr_double_t d) |
| vector | operator% (const nr_complex_t z, vector v) |
| vector | operator% (const nr_double_t d, vector v) |
| vector | operator% (vector v1, vector v2) |
| vector | linspace (nr_double_t start, nr_double_t stop, int points) |
| vector | logspace (nr_double_t start, nr_double_t stop, int points) |
| vector | cumsum (vector v) |
| vector | cumavg (vector v) |
| vector | cumprod (vector v) |
| vector | ceil (vector v) |
| vector | fix (vector v) |
| vector | floor (vector v) |
| vector | round (vector v) |
| vector | sqr (vector v) |
| vector | step (vector v) |
| static nr_double_t | integrate_n (vector v) |
| vector | erf (vector v) |
| vector | erfc (vector v) |
| vector | erfinv (vector v) |
| vector | erfcinv (vector v) |
| vector | rad2deg (vector v) |
| vector | deg2rad (vector v) |
| vector | i0 (vector v) |
| vector | jn (const int n, vector v) |
| vector | yn (const int n, vector v) |
| vector | polar (const nr_complex_t a, vector v) |
| vector | polar (vector v, const nr_complex_t p) |
| vector | polar (vector a, vector p) |
| vector | atan2 (const nr_double_t y, vector v) |
| vector | atan2 (vector v, const nr_double_t x) |
| vector | atan2 (vector y, vector x) |
| vector | w2dbm (vector v) |
| vector | dbm2w (vector v) |
| nr_double_t | integrate (vector v, const nr_double_t h) |
| nr_complex_t | integrate (vector v, const nr_complex_t h) |
| vector | dbm (vector v, const nr_complex_t z) |
| vector | runavg (const nr_complex_t x, const int n) |
| vector | runavg (vector v, const int n) |
| qucs::vector | runavg (qucs::vector, const int) |
Detailed Description
It is prefered to add all used funcions into the qucs namespace. Doing so one is forced do think about compatibility instead of using std directly. Inline is optional at this moment
- Todo:
- test if inline indeed performace improves (optimization flags should inline them anyway)
Function Documentation
| vector qucs::abs | ( | vector | v | ) |
Definition at line 337 of file vector.cpp.
| nr_double_t qucs::abs | ( | const nr_double_t | r | ) |
| matvec qucs::abs | ( | matvec | a | ) |
Definition at line 517 of file matvec.cpp.
| matrix qucs::abs | ( | matrix | a | ) |
Computes magnitude of each matrix element.
- Parameters:
-
[in] a matrix
- Todo:
add abs in place
a is const
Definition at line 531 of file matrix.cpp.
| nr_double_t qucs::acos | ( | const nr_double_t | arg | ) |
| nr_complex_t qucs::acos | ( | const nr_complex_t | z | ) |
Compute complex arc cosine.
- Parameters:
-
[in] z complex arc
- Returns:
- arc cosine of z
Definition at line 84 of file complex.cpp.
| vector qucs::acos | ( | vector | v | ) |
Definition at line 469 of file vector.cpp.
| nr_double_t qucs::acosh | ( | const nr_double_t | arg | ) |
| nr_complex_t qucs::acosh | ( | const nr_complex_t | z | ) |
Compute complex arc hyperbolic cosine.
- Parameters:
-
[in] z complex arc
- Returns:
- arc hyperbolic cosine of z
Definition at line 162 of file complex.cpp.
| vector qucs::acosh | ( | vector | v | ) |
Definition at line 535 of file vector.cpp.
| nr_complex_t qucs::acot | ( | const nr_complex_t | z | ) |
Compute complex arc cotangent.
- Parameters:
-
[in] z complex arc
- Returns:
- arc cotangent of z
Definition at line 310 of file complex.cpp.
| vector qucs::acot | ( | vector | v | ) |
Definition at line 499 of file vector.cpp.
| nr_complex_t qucs::acoth | ( | const nr_complex_t | z | ) |
Compute complex argument hyperbolic cotangent.
- Parameters:
-
[in] z complex arc
- Returns:
- argument hyperbolic cotangent of z
Definition at line 332 of file complex.cpp.
| vector qucs::acoth | ( | vector | v | ) |
Definition at line 565 of file vector.cpp.
| matrix qucs::adjoint | ( | matrix | a | ) |
adjoint matrix
The function returns the adjoint complex matrix. This is also called the adjugate or transpose conjugate.
- Parameters:
-
[in] a Matrix to transpose
- Todo:
add adjoint in place
Do not lazy and avoid conj and transpose copy
a is const
Definition at line 522 of file matrix.cpp.
| matvec qucs::adjoint | ( | matvec | a | ) |
Definition at line 553 of file matvec.cpp.
| vector qucs::arg | ( | vector | v | ) |
Definition at line 349 of file vector.cpp.
| matvec qucs::arg | ( | matvec | a | ) |
Definition at line 531 of file matvec.cpp.
| matrix qucs::arg | ( | matrix | a | ) |
Computes the argument of each matrix element.
- Parameters:
-
[in] a matrix
- Todo:
add arg in place
a is const
Definition at line 555 of file matrix.cpp.
| nr_complex_t qucs::asech | ( | const nr_complex_t | z | ) |
Compute complex argument hyperbolic secant.
- Parameters:
-
[in] z complex arc
- Returns:
- argument hyperbolic secant of z
- Todo:
- for symetry reason implement sech
Definition at line 354 of file complex.cpp.
| vector qucs::asech | ( | vector | v | ) |
Definition at line 541 of file vector.cpp.
| nr_double_t qucs::asin | ( | const nr_double_t | arg | ) |
| nr_complex_t qucs::asin | ( | const nr_complex_t | z | ) |
Compute complex arc sine.
- Parameters:
-
[in] z complex arc
- Returns:
- arc sine of z
Definition at line 102 of file complex.cpp.
| vector qucs::asin | ( | vector | v | ) |
Definition at line 463 of file vector.cpp.
| nr_double_t qucs::asinh | ( | const nr_double_t | arg | ) |
| nr_complex_t qucs::asinh | ( | const nr_complex_t | z | ) |
Compute complex arc hyperbolic sine.
- Parameters:
-
[in] z complex arc
- Returns:
- arc hyperbolic sine of z
Definition at line 175 of file complex.cpp.
| vector qucs::asinh | ( | vector | v | ) |
Definition at line 511 of file vector.cpp.
| nr_double_t qucs::atan | ( | const nr_double_t | arg | ) |
| nr_complex_t qucs::atan | ( | const nr_complex_t | z | ) |
Compute complex arc tangent.
- Parameters:
-
[in] z complex arc
- Returns:
- arc tangent of z
Definition at line 117 of file complex.cpp.
| vector qucs::atan | ( | vector | v | ) |
Definition at line 487 of file vector.cpp.
| nr_double_t qucs::atan2 | ( | const nr_double_t | x, |
| const nr_double_t | y | ||
| ) |
| nr_complex_t qucs::atan2 | ( | const nr_complex_t | y, |
| const nr_complex_t | x | ||
| ) |
Compute complex arc tangent fortran like function.
atan2 is a two-argument function that computes the arc tangent of y / x given y and x, but with a range of ![$(-\pi;\pi]$](form_62.png)
- Parameters:
-
[in] z complex angle
- Returns:
- arc tangent of z
Definition at line 377 of file complex.cpp.
| vector qucs::atan2 | ( | const nr_double_t | y, |
| vector | v | ||
| ) |
Definition at line 1176 of file vector.cpp.
| vector qucs::atan2 | ( | vector | v, |
| const nr_double_t | x | ||
| ) |
Definition at line 1183 of file vector.cpp.
| vector qucs::atan2 | ( | vector | y, |
| vector | x | ||
| ) |
Definition at line 1190 of file vector.cpp.
| nr_double_t qucs::atanh | ( | const nr_double_t | arg | ) |
| nr_complex_t qucs::atanh | ( | const nr_complex_t | z | ) |
Compute complex arc hyperbolic tangent.
- Parameters:
-
[in] z complex arc
- Returns:
- arc hyperbolic tangent of z
Definition at line 188 of file complex.cpp.
| vector qucs::atanh | ( | vector | v | ) |
Definition at line 553 of file vector.cpp.
| matrix qucs::atos | ( | matrix | a, |
| nr_complex_t | z1, | ||
| nr_complex_t | z2 | ||
| ) |
Converts chain matrix to scattering parameters.
Converts chain matrix to scattering parameters Formulae are given by [5] and are remembered here:
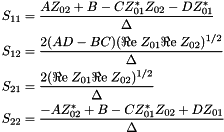
Where:
![\[ \Delta =AZ_{02}+B+CZ_{01}Z_{02}-DZ_{01} \]](form_99.png)
- Parameters:
-
[in] a Chain matrix [in] z1 impedance at input 1 [in] z2 impedance at input 2
- Returns:
- Scattering matrix
- Bug:
- Do not use fabs
- Todo:
- a, z1, z2 const
Definition at line 1222 of file matrix.cpp.
| nr_complex_t qucs::avg | ( | vector | v | ) |
Definition at line 272 of file vector.cpp.
| nr_double_t qucs::b1 | ( | matrix | ) |
| qucs::vector qucs::b1 | ( | matvec | m | ) |
Definition at line 680 of file matvec.cpp.
| nr_complex_t qucs::cbesselj | ( | unsigned int | n, |
| nr_complex_t | z | ||
| ) |
Main entry point for besselj function.
| static nr_complex_t qucs::cbesselj_largearg | ( | unsigned int | n, |
| nr_complex_t | z | ||
| ) | [static] |
| static nr_complex_t qucs::cbesselj_mediumarg | ( | unsigned int | n, |
| nr_complex_t | z | ||
| ) | [static] |
Definition at line 203 of file complex.cpp.
| static nr_complex_t qucs::cbesselj_mediumarg_even | ( | unsigned int | n, |
| nr_complex_t | z | ||
| ) | [static] |
Definition at line 175 of file complex.cpp.
| static nr_complex_t qucs::cbesselj_mediumarg_odd | ( | unsigned int | n, |
| nr_complex_t | z | ||
| ) | [static] |
Definition at line 148 of file complex.cpp.
| static nr_complex_t qucs::cbesselj_smallarg | ( | unsigned int | n, |
| nr_complex_t | z | ||
| ) | [static] |
- Todo:
- Not really adapted to high order therefore we do not check overflow for n >> 1
- Parameters:
-
[in] n order [in] arg arguments
Definition at line 108 of file complex.cpp.
| nr_double_t qucs::ceil | ( | nr_double_t | arg | ) |
| nr_complex_t qucs::ceil | ( | const nr_complex_t | z | ) |
Complex ceil Ceil is the smallest integral value not less than argument Apply ceil to real and imaginary part.
- Parameters:
-
[in] z complex number
- Returns:
- ceilled complex number
Definition at line 634 of file complex.cpp.
| vector qucs::ceil | ( | vector | v | ) |
Definition at line 1031 of file vector.cpp.
| static nr_double_t qucs::celsius2kelvin | ( | const nr_double_t | x | ) | [inline, static] |
| nr_complex_t qucs::cofactor | ( | matrix | a, |
| int | u, | ||
| int | v | ||
| ) |
Computes the complex cofactor of the given determinant.
The cofactor is the determinant obtained by deleting the row and column of a given element of a matrix or determinant. The cofactor is preceded by a + or - sign depending of the sign of 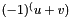
- Bug:
- This algortihm is recursive! Stack overfull!
- Todo:
((u + v) & 1) is cryptic use (u + v)% 2
#ifdef 0
static?
Definition at line 657 of file matrix.cpp.
| nr_type_t qucs::cond_conj | ( | nr_type_t | t | ) | [inline] |
Definition at line 871 of file eqnsys.cpp.
| double qucs::cond_conj | ( | double | t | ) | [inline] |
Definition at line 876 of file eqnsys.cpp.
| tvector<nr_type_t> qucs::conj | ( | tvector< nr_type_t > | a | ) |
Definition at line 295 of file tvector.cpp.
| vector qucs::conj | ( | vector | v | ) |
Definition at line 367 of file vector.cpp.
| matrix qucs::conj | ( | matrix | a | ) |
Conjugate complex matrix.
- Parameters:
-
[in] a Matrix to conjugate
- Todo:
add conj in place
a is const
Definition at line 505 of file matrix.cpp.
| nr_double_t qucs::conj | ( | const nr_double_t | r | ) |
| matvec qucs::conj | ( | matvec | a | ) |
Definition at line 510 of file matvec.cpp.
| nr_double_t qucs::cos | ( | const nr_double_t | arg | ) |
| nr_complex_t qucs::cos | ( | const nr_complex_t | z | ) |
Compute complex cosine.
- Parameters:
-
[in] z complex angle
- Returns:
- cosine of z
Definition at line 57 of file complex.cpp.
| vector qucs::cos | ( | vector | v | ) |
Definition at line 475 of file vector.cpp.
| nr_double_t qucs::cosech | ( | const nr_double_t | d | ) |
| nr_complex_t qucs::cosech | ( | const nr_complex_t | z | ) |
Compute complex argument hyperbolic cosec.
- Parameters:
-
[in] z complex arc
- Returns:
- argument hyperbolic cosec of z
Definition at line 364 of file complex.cpp.
| vector qucs::cosech | ( | vector | v | ) |
Definition at line 529 of file vector.cpp.
| nr_double_t qucs::cosh | ( | const nr_double_t | arg | ) |
| nr_complex_t qucs::cosh | ( | const nr_complex_t | z | ) |
Compute complex hyperbolic cosine.
- Parameters:
-
[in] z complex arc
- Returns:
- hyperbolic cosine of z
Definition at line 135 of file complex.cpp.
| vector qucs::cosh | ( | vector | v | ) |
Definition at line 517 of file vector.cpp.
| nr_complex_t qucs::cot | ( | const nr_complex_t | z | ) |
Compute complex cotangent.
- Parameters:
-
[in] z complex angle
- Returns:
- cotangent of z
Definition at line 298 of file complex.cpp.
| vector qucs::cot | ( | vector | v | ) |
Definition at line 493 of file vector.cpp.
| nr_double_t qucs::coth | ( | const nr_double_t | d | ) |
| nr_complex_t qucs::coth | ( | const nr_complex_t | z | ) |
Compute complex hyperbolic cotangent.
- Parameters:
-
[in] z complex angle
- Returns:
- hyperbolic cotangent of z
Definition at line 320 of file complex.cpp.
| vector qucs::coth | ( | vector | v | ) |
Definition at line 559 of file vector.cpp.
| static char* qucs::Cplx2String | ( | nr_complex_t | c | ) | [static] |
Definition at line 163 of file equation.cpp.
| matrix qucs::cstocy | ( | matrix | cs, |
| matrix | y | ||
| ) |
Converts S-parameter noise correlation matrix to admittance noise correlation matrix.
According to [7] fig 2:
![\[ C_y=(I+Y)C_s(I+Y)^+ \]](form_110.png)
Where 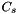 is the scattering noise correlation matrix,
is the scattering noise correlation matrix,  the admittance noise correlation matrix,
the admittance noise correlation matrix, 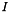 the identity matrix and
the identity matrix and 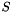 the scattering matrix of device.
the scattering matrix of device. 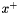 is the adjoint of
is the adjoint of 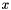
- Warning:
- cs matrix and y matrix are assumed to be normalized
- Parameters:
-
[in] cs S parameter noise correlation [in] y Admittance matrix of device
- Returns:
- admittance noise correlation matrix
- Todo:
- cs, y const
Definition at line 1430 of file matrix.cpp.
| matrix qucs::cstocz | ( | matrix | cs, |
| matrix | z | ||
| ) |
Converts S-parameter noise correlation matrix to impedance noise correlation matrix.
According to [7] fig 2:
![\[ C_z=(I+Z)C_s(I+Z)^+ \]](form_113.png)
Where 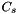 is the scattering noise correlation matrix,
is the scattering noise correlation matrix,  the impedance noise correlation matrix,
the impedance noise correlation matrix, 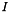 the identity matrix and
the identity matrix and 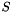 the scattering matrix of device.
the scattering matrix of device. 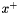 is the adjoint of
is the adjoint of 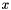
- Warning:
- cs matrix and y matrix are assumed to be normalized
- Parameters:
-
[in] cs S parameter noise correlation [in] z Impedance matrix of device
- Returns:
- Impedance noise correlation matrix
- Todo:
- cs, z const
Definition at line 1483 of file matrix.cpp.
| static nr_double_t qucs::cubic | ( | const nr_double_t | x | ) | [inline, static] |
| vector qucs::cumavg | ( | vector | v | ) |
Definition at line 1011 of file vector.cpp.
| vector qucs::cumprod | ( | vector | v | ) |
Definition at line 1021 of file vector.cpp.
| vector qucs::cumsum | ( | vector | v | ) |
Definition at line 1001 of file vector.cpp.
| matrix qucs::cytocs | ( | matrix | cy, |
| matrix | s | ||
| ) |
Admittance noise correlation matrix to S-parameter noise correlation matrix.
Converts admittance noise correlation matrix to S-parameter noise correlation matrix. According to [7] fig 2:
![\[ C_s=\frac{1}{4}(I+S)C_y(I+S)^+ \]](form_106.png)
Where 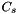 is the scattering noise correlation matrix,
is the scattering noise correlation matrix,  the admittance noise correlation matrix,
the admittance noise correlation matrix, 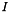 the identity matrix and
the identity matrix and 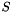 the scattering matrix of device.
the scattering matrix of device. 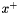 is the adjoint of
is the adjoint of 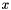
- Warning:
- cy matrix and s matrix are assumed to be normalized
- Parameters:
-
[in] cy Admittance noise correlation [in] s S parameter matrix of device
- Returns:
- S-parameter noise correlation matrix
- Note:
- Assert compatiblity of matrix
- Todo:
- cy s const
Definition at line 1404 of file matrix.cpp.
| matrix qucs::cytocz | ( | matrix | cy, |
| matrix | z | ||
| ) |
Converts admittance noise correlation matrix to impedance noise correlation matrix.
According to [7] fig 2:
![\[ C_z=ZC_yZ^+ \]](form_115.png)
Where  is the impedance correlation matrix,
is the impedance correlation matrix,  the identity matrix and
the identity matrix and  the admittance noise correlation matrix.
the admittance noise correlation matrix. 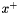 is the adjoint of
is the adjoint of 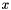
- Warning:
- cy matrix and z matrix are assumed to be normalized
- Parameters:
-
[in] cy Admittance noise correlation [in] z Impedance matrix of device
- Returns:
- Impedance noise correlation matrix
- Todo:
- cs, z const
Definition at line 1531 of file matrix.cpp.
| matrix qucs::cztocs | ( | matrix | cz, |
| matrix | s | ||
| ) |
Converts impedance noise correlation matrix to S-parameter noise correlation matrix.
According to [7] fig 2:
![\[ C_s=\frac{1}{4}(I-S)C_z(I-S) \]](form_111.png)
Where 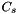 is the scattering noise correlation matrix,
is the scattering noise correlation matrix,  the impedance noise correlation matrix,
the impedance noise correlation matrix, 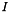 the identity matrix and
the identity matrix and 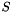 the scattering matrix of device.
the scattering matrix of device. 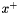 is the adjoint of
is the adjoint of 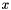
- Warning:
- Cz matrix and s matrix are assumed to be normalized
- Parameters:
-
[in] cz Impedance noise correlation [in] s S parameter matrix of device
- Returns:
- S-parameter noise correlation matrix
- Note:
- Assert compatiblity of matrix
- Todo:
- cz, s const
Definition at line 1457 of file matrix.cpp.
| matrix qucs::cztocy | ( | matrix | cz, |
| matrix | y | ||
| ) |
Converts impedance noise correlation matrix to admittance noise correlation matrix.
According to [7] fig 2:
![\[ C_y=YC_zY^+ \]](form_114.png)
Where  is the impedance correlation matrix,
is the impedance correlation matrix, 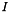 the identity matrix and
the identity matrix and  the admittance noise correlation matrix.
the admittance noise correlation matrix. 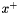 is the adjoint of
is the adjoint of 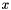
- Warning:
- cz matrix and y matrix are assumed to be normalized
- Parameters:
-
[in] cz impedance noise correlation [in] y Admittance matrix of device
- Returns:
- admittance noise correlation matrix
- Todo:
- cs, y const
Definition at line 1507 of file matrix.cpp.
| vector qucs::dB | ( | vector | v | ) |
Definition at line 373 of file vector.cpp.
| matvec qucs::dB | ( | matvec | a | ) |
Definition at line 524 of file matvec.cpp.
| nr_double_t qucs::dB | ( | const nr_complex_t | z | ) |
Magnitude in dB Compute 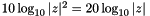 .
.
- Parameters:
-
[in] z complex number
- Returns:
- Magnitude in dB
Definition at line 528 of file complex.cpp.
| matrix qucs::dB | ( | matrix | a | ) |
Computes magnitude in dB of each matrix element.
- Parameters:
-
[in] a matrix
Definition at line 542 of file matrix.cpp.
| vector qucs::dbm | ( | vector | v, |
| const nr_complex_t | z | ||
| ) |
Definition at line 1236 of file vector.cpp.
| vector qucs::dbm2w | ( | vector | v | ) |
Definition at line 1214 of file vector.cpp.
| matrix qucs::deg2rad | ( | matrix | ) |
| nr_double_t qucs::deg2rad | ( | const nr_double_t | x | ) |
| matvec qucs::deg2rad | ( | matvec | a | ) |
Definition at line 693 of file matvec.cpp.
| nr_double_t qucs::deg2rad | ( | const nr_complex_t | x | ) |
rad2deg Convert radian to degree
- Parameters:
-
x input
- Returns:
- real(x)*pi/180
Definition at line 916 of file complex.cpp.
| vector qucs::deg2rad | ( | vector | v | ) |
Definition at line 1123 of file vector.cpp.
| qucs::vector qucs::det | ( | matvec | a | ) |
Definition at line 475 of file matvec.cpp.
| nr_complex_t qucs::det | ( | matrix | a | ) |
Compute determinant of the given matrix.
- Parameters:
-
[in] a matrix
- Returns:
- Complex determinant
- Todo:
- a const?
Definition at line 762 of file matrix.cpp.
| nr_complex_t qucs::detGauss | ( | matrix | a | ) |
Compute determinant Gaussian algorithm.
Compute determinant of the given matrix using the Gaussian algorithm. This means to triangulate the matrix and multiply all the diagonal elements.
- Parameters:
-
[in] a matrix
- Note:
- assert square matrix
- Todo:
static ?
a const?
Definition at line 717 of file matrix.cpp.
| nr_complex_t qucs::detLaplace | ( | matrix | a | ) |
Compute determinant of the given matrix using Laplace expansion.
The Laplace expansion of the determinant of an n by n square matrix a expresses the determinant of a as a sum of n determinants of (n-1) by (n-1) sub-matrices of a. There are 2n such expressions, one for each row and column of a.
See Wikipedia http://en.wikipedia.org/wiki/Laplace_expansion
- Parameters:
-
[in] a matrix
- Bug:
- This algortihm is recursive! Stack overfull!
- Note:
- assert square matrix
- Todo:
#ifdef 0
static ?
Definition at line 686 of file matrix.cpp.
| matrix qucs::diagonal | ( | vector | ) |
| matrix qucs::diagonal | ( | qucs::vector | diag | ) |
Create a diagonal matrix from a vector.
- Parameters:
-
[in] diag vector to write on the diagonal
- Todo:
- diag is const
Definition at line 624 of file matrix.cpp.
| vector qucs::diff | ( | vector | var, |
| vector | dep, | ||
| int | n | ||
| ) |
Definition at line 600 of file vector.cpp.
| nr_double_t qucs::erf | ( | nr_double_t | arg | ) |
| nr_complex_t qucs::erf | ( | const nr_complex_t | z | ) |
Error function.
- Parameters:
-
[in] z argument
- Returns:
- Error function
- Bug:
- Not implemented
Definition at line 766 of file complex.cpp.
| vector qucs::erf | ( | vector | v | ) |
Definition at line 1093 of file vector.cpp.
| nr_complex_t qucs::erfc | ( | const nr_complex_t | z | ) |
Complementart error function.
- Parameters:
-
[in] z argument
- Returns:
- Complementary error function
- Bug:
- Not implemented
Definition at line 784 of file complex.cpp.
| vector qucs::erfc | ( | vector | v | ) |
Definition at line 1099 of file vector.cpp.
| nr_complex_t qucs::erfcinv | ( | const nr_complex_t | z | ) |
Inverse of complementart error function.
- Parameters:
-
[in] z argument
- Returns:
- Inverse of complementary error function
- Bug:
- Not implemented
Definition at line 813 of file complex.cpp.
| vector qucs::erfcinv | ( | vector | v | ) |
Definition at line 1111 of file vector.cpp.
| nr_complex_t qucs::erfinv | ( | const nr_complex_t | z | ) |
Inverse of error function.
- Parameters:
-
[in] z argument
- Returns:
- Inverse of error function
- Bug:
- Not implemented
Definition at line 802 of file complex.cpp.
| vector qucs::erfinv | ( | vector | v | ) |
Definition at line 1105 of file vector.cpp.
| static void qucs::euclidian_update | ( | nr_double_t | a, |
| nr_double_t & | n, | ||
| nr_double_t & | scale | ||
| ) | [inline, static] |
Helper function for the euclidian norm calculators.
Definition at line 830 of file eqnsys.cpp.
| nr_double_t qucs::exp | ( | const nr_double_t | arg | ) |
| nr_complex_t qucs::exp | ( | const nr_complex_t | z | ) |
Compute complex exponential.
- Parameters:
-
[in] z complex number
- Returns:
- exponential of z
Definition at line 205 of file complex.cpp.
| vector qucs::exp | ( | vector | v | ) |
Definition at line 386 of file vector.cpp.
Create identity matrix with specified number of rows and columns.
- Parameters:
-
[in] rs row number [in] cs column number
- Todo:
Avoid res.get*
Use memset
rs, cs are const
Definition at line 603 of file matrix.cpp.
Create a square identity matrix.
- Parameters:
-
[in] s row or column number of square matrix
- Todo:
Do not by lazy and implement it
s is const
Definition at line 616 of file matrix.cpp.
| unsigned int qucs::factorial | ( | unsigned int | n | ) |
| nr_double_t qucs::fix | ( | const nr_double_t | d | ) |
| nr_complex_t qucs::fix | ( | const nr_complex_t | z | ) |
Complex fix.
Apply fix to real and imaginary part
- Parameters:
-
[in] z complex number
- Returns:
- fixed complex number
- Todo:
- why not using real fix
Definition at line 645 of file complex.cpp.
| vector qucs::fix | ( | vector | v | ) |
Definition at line 1037 of file vector.cpp.
| nr_double_t qucs::floor | ( | nr_double_t | arg | ) |
| nr_complex_t qucs::floor | ( | const nr_complex_t | z | ) |
Complex floor.
floor is the largest integral value not greater than argument Apply floor to real and imaginary part
- Parameters:
-
[in] z complex number
- Returns:
- floored complex number
Definition at line 623 of file complex.cpp.
| vector qucs::floor | ( | vector | v | ) |
Definition at line 1043 of file vector.cpp.
| nr_double_t qucs::fmod | ( | nr_double_t | arg | ) |
| nr_complex_t qucs::fmod | ( | const nr_complex_t | x, |
| const nr_complex_t | y | ||
| ) |
Complex fmod Apply fmod to the complex z.
- Parameters:
-
[in] x complex number (numerator) [in] y complex number (denominator)
- Returns:
- return
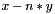 where n is the quotient of
where n is the quotient of 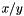 , rounded towards zero to an integer.
, rounded towards zero to an integer.
- Todo:
- add fmod to manual
Definition at line 662 of file complex.cpp.
| static nr_double_t qucs::givens | ( | nr_double_t | a, |
| nr_double_t | b, | ||
| nr_double_t & | c, | ||
| nr_double_t & | s | ||
| ) | [inline, static] |
Helper function computes Givens rotation.
Definition at line 1339 of file eqnsys.cpp.
| matrix qucs::gtos | ( | matrix | g, |
| nr_complex_t | z1, | ||
| nr_complex_t | z2 | ||
| ) |
Definition at line 1355 of file matrix.cpp.
| matrix qucs::htos | ( | matrix | h, |
| nr_complex_t | z1, | ||
| nr_complex_t | z2 | ||
| ) |
Converts hybrid matrix to scattering parameters.
Formulae are given by [5] and are remembered here:
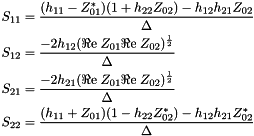
Where  is:
is:
![\[ \Delta=(Z_{01}+h_{11})(1+h_{22}Z_{02})-h_{12}h_{21}Z_{02} \]](form_104.png)
- Parameters:
-
[in] h hybrid matrix [in] z1 impedance at input 1 [in] z2 impedance at input 2
- Returns:
- scattering matrix
- Note:
- Assert 2 by 2 matrix
- Todo:
- Why not h,z1,z2 const
Definition at line 1307 of file matrix.cpp.
| nr_complex_t qucs::i0 | ( | const nr_complex_t | z | ) |
Modified Bessel function of first kind.
- Parameters:
-
[in] z argument
- Returns:
- Modified Bessel function of first kind of order 0
- Bug:
- Not implemented
Definition at line 754 of file complex.cpp.
| vector qucs::i0 | ( | vector | v | ) |
Definition at line 1129 of file vector.cpp.
| vector qucs::imag | ( | vector | v | ) |
Definition at line 361 of file vector.cpp.
| nr_double_t qucs::imag | ( | const nr_double_t | r | ) |
| matvec qucs::imag | ( | matvec | a | ) |
Definition at line 545 of file matvec.cpp.
| matrix qucs::imag | ( | matrix | a | ) |
Imaginary part matrix.
- Parameters:
-
[in] a matrix
- Todo:
add imag in place
a is const
Definition at line 581 of file matrix.cpp.
| static int qucs::insfunc | ( | struct nodelist_t * | n1, |
| struct nodelist_t * | n2 | ||
| ) | [static] |
Definition at line 197 of file nodelist.cpp.
| nr_double_t qucs::integrate | ( | vector | v, |
| const nr_double_t | h | ||
| ) |
Definition at line 1221 of file vector.cpp.
| nr_complex_t qucs::integrate | ( | vector | v, |
| const nr_complex_t | h | ||
| ) |
Definition at line 1228 of file vector.cpp.
| static nr_double_t qucs::integrate_n | ( | vector | v | ) | [static] |
Definition at line 1067 of file vector.cpp.
| tmatrix<nr_type_t> qucs::inverse | ( | tmatrix< nr_type_t > | a | ) |
Definition at line 197 of file tmatrix.cpp.
| matvec qucs::inverse | ( | matvec | a | ) |
Definition at line 482 of file matvec.cpp.
| matrix qucs::inverse | ( | matrix | a | ) |
Compute inverse matrix.
- Parameters:
-
[in] a matrix to invert
- Todo:
- a is const
Definition at line 847 of file matrix.cpp.
| matrix qucs::inverseGaussJordan | ( | matrix | a | ) |
Compute inverse matrix using Gauss-Jordan elimination.
Compute inverse matrix of the given matrix by Gauss-Jordan elimination.
- Todo:
a const?
static?
- Note:
- assert non singulat matix
- Parameters:
-
[in] a matrix to invert
Definition at line 798 of file matrix.cpp.
| matrix qucs::inverseLaplace | ( | matrix | a | ) |
Compute inverse matrix using Laplace expansion.
Compute inverse matrix of the given matrix using Laplace expansion.
- Parameters:
-
[in] a matrix to invert
- Todo:
Static?
#ifdef 0
- Bug:
- recursive! Stack overflow
- Todo:
- a const?
Definition at line 779 of file matrix.cpp.
| nr_complex_t qucs::jn | ( | const int | n, |
| const nr_complex_t | z | ||
| ) |
Bessel function of first kind.
- Parameters:
-
[in] n order [in] z argument
- Returns:
- Bessel function of first kind of order n
- Bug:
- Not implemented
Definition at line 729 of file complex.cpp.
Definition at line 1135 of file vector.cpp.
| static nr_double_t qucs::kelvin2celsius | ( | const nr_double_t | x | ) | [inline, static] |
| nr_double_t qucs::limexp | ( | const nr_double_t | r | ) |
| vector qucs::limexp | ( | vector | v | ) |
Definition at line 392 of file vector.cpp.
| nr_complex_t qucs::limexp | ( | const nr_complex_t | z | ) |
Compute limited complex exponential.
- Parameters:
-
[in] z complex number
- Returns:
- limited exponential of z
- Todo:
- Change limexp(real) limexp(complex) file order
Definition at line 539 of file complex.cpp.
| vector qucs::linspace | ( | nr_double_t | start, |
| nr_double_t | stop, | ||
| int | points | ||
| ) |
Definition at line 959 of file vector.cpp.
| nr_double_t qucs::log | ( | const nr_double_t | arg | ) |
| nr_complex_t qucs::log | ( | const nr_complex_t | z | ) |
Compute principal value of natural logarithm of z.
- Parameters:
-
[in] z complex number
- Returns:
- principal value of natural logarithm of z
Definition at line 215 of file complex.cpp.
| vector qucs::log | ( | vector | v | ) |
Definition at line 398 of file vector.cpp.
| nr_double_t qucs::log10 | ( | const nr_double_t | arg | ) |
| nr_complex_t qucs::log10 | ( | const nr_complex_t | z | ) |
Compute principal value of decimal logarithm of z.
- Parameters:
-
[in] z complex number
- Returns:
- principal value of decimal logarithm of z
Definition at line 225 of file complex.cpp.
| vector qucs::log10 | ( | vector | v | ) |
Definition at line 404 of file vector.cpp.
| nr_complex_t qucs::log2 | ( | const nr_complex_t | z | ) |
Compute principal value of binary logarithm of z.
- Parameters:
-
[in] z complex number
- Returns:
- principal value of binary logarithm of z
Definition at line 393 of file complex.cpp.
| vector qucs::log2 | ( | vector | v | ) |
Definition at line 410 of file vector.cpp.
| vector qucs::logspace | ( | nr_double_t | start, |
| nr_double_t | stop, | ||
| int | points | ||
| ) |
Definition at line 974 of file vector.cpp.
| nr_double_t qucs::maxnorm | ( | tvector< nr_type_t > | a | ) |
Definition at line 284 of file tvector.cpp.
| nr_double_t qucs::norm | ( | tvector< nr_type_t > | a | ) |
Definition at line 245 of file tvector.cpp.
| nr_double_t qucs::norm | ( | const nr_complex_t | z | ) |
Compute euclidian norm of complex number.
Compute 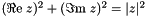
- Parameters:
-
[in] z Complex number
- Returns:
- Euclidian norm of z
Definition at line 283 of file complex.cpp.
| vector qucs::norm | ( | vector | v | ) |
Definition at line 343 of file vector.cpp.
| nr_double_t qucs::norm | ( | const nr_double_t | r | ) |
| bool qucs::operator!= | ( | const nr_complex_t | z1, |
| const nr_complex_t | z2 | ||
| ) |
Inequality of two complex.
- Todo:
- Why not inline
- Note:
- Like inequality of double this test is meaningless in finite precision Use instead fabs(x-x0) > tol
Definition at line 865 of file complex.cpp.
| nr_complex_t qucs::operator% | ( | const nr_complex_t | z1, |
| const nr_complex_t | z2 | ||
| ) |
| nr_complex_t qucs::operator% | ( | const nr_complex_t | z1, |
| const nr_double_t | r2 | ||
| ) |
| nr_complex_t qucs::operator% | ( | const nr_double_t | r1, |
| const nr_complex_t | z2 | ||
| ) |
| vector qucs::operator% | ( | vector | v, |
| const nr_complex_t | z | ||
| ) |
Definition at line 866 of file vector.cpp.
| vector qucs::operator% | ( | vector | v, |
| const nr_double_t | d | ||
| ) |
Definition at line 873 of file vector.cpp.
| vector qucs::operator% | ( | const nr_complex_t | z, |
| vector | v | ||
| ) |
Definition at line 880 of file vector.cpp.
| vector qucs::operator% | ( | const nr_double_t | d, |
| vector | v | ||
| ) |
Definition at line 887 of file vector.cpp.
| vector qucs::operator% | ( | vector | v1, |
| vector | v2 | ||
| ) |
Definition at line 894 of file vector.cpp.
| tvector<nr_type_t> qucs::operator* | ( | nr_double_t | s, |
| tvector< nr_type_t > | a | ||
| ) |
Definition at line 156 of file tvector.cpp.
| tvector<nr_type_t> qucs::operator* | ( | tvector< nr_type_t > | a, |
| nr_double_t | s | ||
| ) |
Definition at line 164 of file tvector.cpp.
| tvector<nr_type_t> qucs::operator* | ( | tvector< nr_type_t > | a, |
| tvector< nr_type_t > | b | ||
| ) |
Definition at line 170 of file tvector.cpp.
| tmatrix<nr_type_t> qucs::operator* | ( | tmatrix< nr_type_t > | a, |
| tmatrix< nr_type_t > | b | ||
| ) |
Definition at line 275 of file tmatrix.cpp.
| tvector<nr_type_t> qucs::operator* | ( | tmatrix< nr_type_t > | a, |
| tvector< nr_type_t > | b | ||
| ) |
Definition at line 291 of file tmatrix.cpp.
| matrix qucs::operator* | ( | matrix | a, |
| nr_complex_t | z | ||
| ) |
Matrix scaling complex version.
- Parameters:
-
[in] a matrix to scale [in] z scaling complex
- Returns:
- Scaled matrix
- Todo:
- Why not a and z const
Definition at line 298 of file matrix.cpp.
| tvector<nr_type_t> qucs::operator* | ( | tvector< nr_type_t > | a, |
| tmatrix< nr_type_t > | b | ||
| ) |
Definition at line 306 of file tmatrix.cpp.
| matrix qucs::operator* | ( | nr_complex_t | z, |
| matrix | a | ||
| ) |
Matrix scaling complex version (different order)
- Parameters:
-
[in] a matrix to scale [in] z scaling complex
- Returns:
- Scaled matrix
- Todo:
Why not a and z const
Why not inline
Definition at line 313 of file matrix.cpp.
| matrix qucs::operator* | ( | matrix | a, |
| nr_double_t | d | ||
| ) |
Matrix scaling complex version.
- Parameters:
-
[in] a matrix to scale [in] d scaling real
- Returns:
- Scaled matrix
- Todo:
- Why not d and a const
Definition at line 323 of file matrix.cpp.
| matrix qucs::operator* | ( | nr_double_t | d, |
| matrix | a | ||
| ) |
Matrix scaling real version (different order)
- Parameters:
-
[in] a matrix to scale [in] d scaling real
- Returns:
- Scaled matrix
- Todo:
Why not inline?
Why not d and a const
Definition at line 338 of file matrix.cpp.
| matrix qucs::operator* | ( | matrix | a, |
| matrix | b | ||
| ) |
Matrix multiplication.
Dumb and not optimized matrix multiplication
- Parameters:
-
a] first matrix b] second matrix
- Note:
- assert compatibility
- Todo:
- a and b are const
Definition at line 378 of file matrix.cpp.
| matvec qucs::operator* | ( | matvec | a, |
| nr_complex_t | z | ||
| ) |
Definition at line 395 of file matvec.cpp.
| matvec qucs::operator* | ( | nr_complex_t | z, |
| matvec | a | ||
| ) |
Definition at line 402 of file matvec.cpp.
| matvec qucs::operator* | ( | matvec | a, |
| nr_double_t | d | ||
| ) |
Definition at line 407 of file matvec.cpp.
| matvec qucs::operator* | ( | nr_double_t | d, |
| matvec | a | ||
| ) |
Definition at line 414 of file matvec.cpp.
| matvec qucs::operator* | ( | matvec | a, |
| qucs::vector | b | ||
| ) |
Definition at line 419 of file matvec.cpp.
| matvec qucs::operator* | ( | qucs::vector | a, |
| matvec | b | ||
| ) |
Definition at line 427 of file matvec.cpp.
| matvec qucs::operator* | ( | matvec | a, |
| matvec | b | ||
| ) |
Definition at line 454 of file matvec.cpp.
| matvec qucs::operator* | ( | matvec | a, |
| matrix | b | ||
| ) |
Definition at line 462 of file matvec.cpp.
| matvec qucs::operator* | ( | matrix | a, |
| matvec | b | ||
| ) |
Definition at line 470 of file matvec.cpp.
| vector qucs::operator* | ( | vector | v1, |
| vector | v2 | ||
| ) |
Definition at line 775 of file vector.cpp.
| vector qucs::operator* | ( | vector | v, |
| const nr_complex_t | c | ||
| ) |
Definition at line 788 of file vector.cpp.
| vector qucs::operator* | ( | vector | v, |
| const nr_double_t | d | ||
| ) |
Definition at line 794 of file vector.cpp.
| vector qucs::operator* | ( | const nr_complex_t | c, |
| vector | v | ||
| ) |
Definition at line 800 of file vector.cpp.
| vector qucs::operator* | ( | const nr_double_t | d, |
| vector | v | ||
| ) |
Definition at line 804 of file vector.cpp.
| tvector<nr_type_t> qucs::operator+ | ( | tvector< nr_type_t > | a, |
| tvector< nr_type_t > | b | ||
| ) |
Definition at line 100 of file tvector.cpp.
| matrix qucs::operator+ | ( | matrix | a, |
| matrix | b | ||
| ) |
Matrix addition.
- Parameters:
-
a] first matrix b] second matrix
- Note:
- assert same size
- Todo:
- a and b are const
Definition at line 228 of file matrix.cpp.
| tvector<nr_type_t> qucs::operator+ | ( | nr_type_t | s, |
| tvector< nr_type_t > | a | ||
| ) |
Definition at line 231 of file tvector.cpp.
| tvector<nr_type_t> qucs::operator+ | ( | tvector< nr_type_t > | a, |
| nr_type_t | s | ||
| ) |
Definition at line 239 of file tvector.cpp.
| matvec qucs::operator+ | ( | matvec | a, |
| matvec | b | ||
| ) |
Definition at line 249 of file matvec.cpp.
| matvec qucs::operator+ | ( | matvec | a, |
| matrix | b | ||
| ) |
Definition at line 258 of file matvec.cpp.
| matvec qucs::operator+ | ( | matvec | a, |
| qucs::vector | b | ||
| ) |
Definition at line 266 of file matvec.cpp.
| matvec qucs::operator+ | ( | qucs::vector | b, |
| matvec | a | ||
| ) |
Definition at line 274 of file matvec.cpp.
| matvec qucs::operator+ | ( | matrix | a, |
| matvec | b | ||
| ) |
Definition at line 279 of file matvec.cpp.
| matvec qucs::operator+ | ( | matvec | a, |
| nr_complex_t | z | ||
| ) |
Definition at line 284 of file matvec.cpp.
| matvec qucs::operator+ | ( | nr_complex_t | z, |
| matvec | a | ||
| ) |
Definition at line 291 of file matvec.cpp.
| matvec qucs::operator+ | ( | matvec | a, |
| nr_double_t | d | ||
| ) |
Definition at line 298 of file matvec.cpp.
| matvec qucs::operator+ | ( | nr_double_t | d, |
| matvec | a | ||
| ) |
Definition at line 305 of file matvec.cpp.
| matrix qucs::operator+ | ( | matrix | a, |
| nr_complex_t | z | ||
| ) |
Complex scalar addition.
- Parameters:
-
[in] a matrix [in] z complex to add
- Todo:
Move near other +
a and z are const
Definition at line 399 of file matrix.cpp.
| matrix qucs::operator+ | ( | nr_complex_t | z, |
| matrix | a | ||
| ) |
Complex scalar addition different order.
- Parameters:
-
[in] a matrix [in] z complex to add
- Todo:
Move near other +
a and z are const
Why not inline
Definition at line 414 of file matrix.cpp.
| matrix qucs::operator+ | ( | matrix | a, |
| nr_double_t | d | ||
| ) |
Real scalar addition.
- Parameters:
-
[in] a matrix [in] d real to add
- Todo:
Move near other +
a and d are const
Definition at line 424 of file matrix.cpp.
| matrix qucs::operator+ | ( | nr_double_t | d, |
| matrix | a | ||
| ) |
Real scalar addition different order.
- Parameters:
-
[in] a matrix [in] d real to add
- Todo:
Move near other +
a and d are const
Why not inline
Definition at line 439 of file matrix.cpp.
| vector qucs::operator+ | ( | vector | v1, |
| vector | v2 | ||
| ) |
Definition at line 665 of file vector.cpp.
| vector qucs::operator+ | ( | vector | v, |
| const nr_complex_t | c | ||
| ) |
Definition at line 678 of file vector.cpp.
| vector qucs::operator+ | ( | const nr_complex_t | c, |
| vector | v | ||
| ) |
Definition at line 684 of file vector.cpp.
| vector qucs::operator+ | ( | vector | v, |
| const nr_double_t | d | ||
| ) |
Definition at line 688 of file vector.cpp.
| vector qucs::operator+ | ( | const nr_double_t | d, |
| vector | v | ||
| ) |
Definition at line 694 of file vector.cpp.
| tvector<nr_type_t> qucs::operator- | ( | tvector< nr_type_t > | a, |
| tvector< nr_type_t > | b | ||
| ) |
Definition at line 120 of file tvector.cpp.
| tvector<nr_type_t> qucs::operator- | ( | tvector< nr_type_t > | a | ) |
Definition at line 204 of file tvector.cpp.
| matrix qucs::operator- | ( | matrix | a, |
| matrix | b | ||
| ) |
Matrix subtraction.
- Parameters:
-
a] first matrix b] second matrix
- Note:
- assert same size
- Todo:
- a and b are const
Definition at line 259 of file matrix.cpp.
| matvec qucs::operator- | ( | matvec | a, |
| nr_complex_t | z | ||
| ) |
Definition at line 312 of file matvec.cpp.
| matvec qucs::operator- | ( | nr_complex_t | z, |
| matvec | a | ||
| ) |
Definition at line 319 of file matvec.cpp.
| matvec qucs::operator- | ( | matvec | a, |
| nr_double_t | d | ||
| ) |
Definition at line 326 of file matvec.cpp.
| matvec qucs::operator- | ( | nr_double_t | d, |
| matvec | a | ||
| ) |
Definition at line 333 of file matvec.cpp.
| matvec qucs::operator- | ( | matvec | a, |
| matvec | b | ||
| ) |
Definition at line 348 of file matvec.cpp.
| matvec qucs::operator- | ( | matvec | a, |
| matrix | b | ||
| ) |
Definition at line 357 of file matvec.cpp.
| matvec qucs::operator- | ( | matrix | a, |
| matvec | b | ||
| ) |
Definition at line 365 of file matvec.cpp.
| matvec qucs::operator- | ( | matvec | a, |
| qucs::vector | b | ||
| ) |
Definition at line 370 of file matvec.cpp.
| matvec qucs::operator- | ( | qucs::vector | b, |
| matvec | a | ||
| ) |
Definition at line 375 of file matvec.cpp.
| matrix qucs::operator- | ( | matrix | a, |
| nr_complex_t | z | ||
| ) |
Complex scalar substraction.
- Parameters:
-
[in] a matrix [in] z complex to add
- Todo:
Move near other +
a and z are const
Why not inline
Definition at line 450 of file matrix.cpp.
| matrix qucs::operator- | ( | nr_complex_t | z, |
| matrix | a | ||
| ) |
Complex scalar substraction different order.
- Parameters:
-
[in] a matrix [in] z complex to add
- Todo:
Move near other +
a and z are const
Why not inline
Definition at line 461 of file matrix.cpp.
| matrix qucs::operator- | ( | matrix | a, |
| nr_double_t | d | ||
| ) |
Real scalar substraction.
- Parameters:
-
[in] a matrix [in] z real to add
- Todo:
Move near other +
a and z are const
Why not inline
Definition at line 472 of file matrix.cpp.
| matrix qucs::operator- | ( | nr_double_t | d, |
| matrix | a | ||
| ) |
Real scalar substraction different order.
- Parameters:
-
[in] a matrix [in] z real to add
- Todo:
Move near other +
a and z are const
Why not inline
Definition at line 483 of file matrix.cpp.
| vector qucs::operator- | ( | vector | v1, |
| vector | v2 | ||
| ) |
Definition at line 721 of file vector.cpp.
| vector qucs::operator- | ( | vector | v, |
| const nr_complex_t | c | ||
| ) |
Definition at line 734 of file vector.cpp.
| vector qucs::operator- | ( | vector | v, |
| const nr_double_t | d | ||
| ) |
Definition at line 740 of file vector.cpp.
| vector qucs::operator- | ( | const nr_complex_t | c, |
| vector | v | ||
| ) |
Definition at line 746 of file vector.cpp.
| vector qucs::operator- | ( | const nr_double_t | d, |
| vector | v | ||
| ) |
Definition at line 752 of file vector.cpp.
| matrix qucs::operator/ | ( | matrix | a, |
| nr_complex_t | z | ||
| ) |
Matrix scaling division by complex version.
- Parameters:
-
[in] a matrix to scale [in] z scaling complex
- Returns:
- Scaled matrix
- Todo:
- Why not a and z const
Definition at line 348 of file matrix.cpp.
| matrix qucs::operator/ | ( | matrix | a, |
| nr_double_t | d | ||
| ) |
Matrix scaling division by real version.
- Parameters:
-
[in] a matrix to scale [in] d scaling real
- Returns:
- Scaled matrix
- Todo:
- Why not a and d const
Definition at line 362 of file matrix.cpp.
| matvec qucs::operator/ | ( | matvec | a, |
| nr_complex_t | z | ||
| ) |
Definition at line 432 of file matvec.cpp.
| matvec qucs::operator/ | ( | matvec | a, |
| nr_double_t | d | ||
| ) |
Definition at line 439 of file matvec.cpp.
| matvec qucs::operator/ | ( | matvec | a, |
| qucs::vector | b | ||
| ) |
Definition at line 446 of file matvec.cpp.
| vector qucs::operator/ | ( | vector | v1, |
| vector | v2 | ||
| ) |
Definition at line 825 of file vector.cpp.
| vector qucs::operator/ | ( | vector | v, |
| const nr_complex_t | c | ||
| ) |
Definition at line 840 of file vector.cpp.
| vector qucs::operator/ | ( | vector | v, |
| const nr_double_t | d | ||
| ) |
Definition at line 846 of file vector.cpp.
| vector qucs::operator/ | ( | const nr_complex_t | c, |
| vector | v | ||
| ) |
Definition at line 852 of file vector.cpp.
| vector qucs::operator/ | ( | const nr_double_t | d, |
| vector | v | ||
| ) |
Definition at line 859 of file vector.cpp.
| bool qucs::operator< | ( | tvector< nr_type_t > | a, |
| tvector< nr_type_t > | b | ||
| ) |
Definition at line 213 of file tvector.cpp.
| bool qucs::operator< | ( | const nr_complex_t | z1, |
| const nr_complex_t | z2 | ||
| ) |
| bool qucs::operator<= | ( | const nr_complex_t | z1, |
| const nr_complex_t | z2 | ||
| ) |
| bool qucs::operator== | ( | const nr_complex_t | z1, |
| const nr_complex_t | z2 | ||
| ) |
Equality of two complex.
- Todo:
- Why not inline
- Note:
- Like equality of double this test is meaningless in finite precision Use instead fabs(x-x0) < tol
Definition at line 854 of file complex.cpp.
| bool qucs::operator> | ( | tvector< nr_type_t > | a, |
| tvector< nr_type_t > | b | ||
| ) |
Definition at line 222 of file tvector.cpp.
| bool qucs::operator> | ( | const nr_complex_t | z1, |
| const nr_complex_t | z2 | ||
| ) |
| bool qucs::operator>= | ( | const nr_complex_t | z1, |
| const nr_complex_t | z2 | ||
| ) |
| nr_complex_t qucs::polar | ( | const nr_double_t | mag, |
| const nr_double_t | ang | ||
| ) |
Construct a complex number using polar notation.
- Parameters:
-
[in] mag Magnitude [in] ang Angle
- Returns:
- complex number in rectangular form
Definition at line 551 of file complex.cpp.
| nr_complex_t qucs::polar | ( | const nr_complex_t | a, |
| const nr_complex_t | p | ||
| ) |
Extension of polar construction to complex.
- Parameters:
-
[in] a Magnitude [in] p Angle
- Returns:
- complex number in rectangular form
- Bug:
- Do not seems holomorph form of real polar
- Bug:
- are these needed/used?
Definition at line 566 of file complex.cpp.
| vector qucs::polar | ( | const nr_complex_t | a, |
| vector | v | ||
| ) |
Definition at line 1147 of file vector.cpp.
| vector qucs::polar | ( | vector | v, |
| const nr_complex_t | p | ||
| ) |
Definition at line 1153 of file vector.cpp.
| vector qucs::polar | ( | vector | a, |
| vector | p | ||
| ) |
Definition at line 1159 of file vector.cpp.
| matvec qucs::pow | ( | matvec | , |
| vector | |||
| ) |
| nr_double_t qucs::pow | ( | const nr_double_t | a, |
| const nr_double_t | b | ||
| ) |
| nr_complex_t qucs::pow | ( | const nr_complex_t | z, |
| const nr_double_t | d | ||
| ) |
Compute power function with real exponent.
- Parameters:
-
[in] z complex mantisse [in] d real exponent
- Returns:
- z power d (
 )
)
Definition at line 238 of file complex.cpp.
| nr_complex_t qucs::pow | ( | const nr_double_t | d, |
| const nr_complex_t | z | ||
| ) |
Compute power function with complex exponent but real mantisse.
- Parameters:
-
[in] d real mantisse [in] z complex exponent
- Returns:
- d power z (
 )
)
Definition at line 248 of file complex.cpp.
| nr_complex_t qucs::pow | ( | const nr_complex_t | z1, |
| const nr_complex_t | z2 | ||
| ) |
Compute complex power function.
- Parameters:
-
[in] z1 complex mantisse [in] z2 complex exponent
- Returns:
- d power z (
 )
)
Definition at line 258 of file complex.cpp.
| vector qucs::pow | ( | vector | v, |
| const nr_complex_t | z | ||
| ) |
Definition at line 416 of file vector.cpp.
| vector qucs::pow | ( | vector | v, |
| const nr_double_t | d | ||
| ) |
Definition at line 422 of file vector.cpp.
| vector qucs::pow | ( | const nr_complex_t | z, |
| vector | v | ||
| ) |
Definition at line 428 of file vector.cpp.
| vector qucs::pow | ( | const nr_double_t | d, |
| vector | v | ||
| ) |
Definition at line 434 of file vector.cpp.
| vector qucs::pow | ( | vector | v1, |
| vector | v2 | ||
| ) |
Definition at line 440 of file vector.cpp.
Definition at line 494 of file matvec.cpp.
| matvec qucs::pow | ( | matvec | a, |
| qucs::vector | v | ||
| ) |
Definition at line 501 of file matvec.cpp.
Definition at line 632 of file matrix.cpp.
| nr_complex_t qucs::prod | ( | vector | v | ) |
Definition at line 266 of file vector.cpp.
| nr_double_t qucs::quadr | ( | const nr_double_t | r | ) |
| matrix qucs::rad2deg | ( | matrix | ) |
| nr_double_t qucs::rad2deg | ( | const nr_double_t | x | ) |
| matvec qucs::rad2deg | ( | matvec | a | ) |
Definition at line 687 of file matvec.cpp.
| nr_double_t qucs::rad2deg | ( | const nr_complex_t | x | ) |
rad2deg Convert radian to degree
- Parameters:
-
x input
- Returns:
- real(x)*180/pi
Definition at line 907 of file complex.cpp.
| vector qucs::rad2deg | ( | vector | v | ) |
Definition at line 1117 of file vector.cpp.
| vector qucs::real | ( | vector | v | ) |
Definition at line 355 of file vector.cpp.
| nr_double_t qucs::real | ( | const nr_double_t | r | ) |
| matvec qucs::real | ( | matvec | a | ) |
Definition at line 538 of file matvec.cpp.
| matrix qucs::real | ( | matrix | a | ) |
Real part matrix.
- Parameters:
-
[in] a matrix
- Todo:
add real in place
a is const
Definition at line 568 of file matrix.cpp.
| nr_double_t qucs::rollet | ( | matrix | ) |
| qucs::vector qucs::rollet | ( | matvec | m | ) |
Definition at line 671 of file matvec.cpp.
| nr_double_t qucs::round | ( | nr_double_t | arg | ) |
| nr_complex_t qucs::round | ( | const nr_complex_t | z | ) |
Complex round Round is the nearest integral value Apply round to real and imaginary part.
- Parameters:
-
[in] z complex number
- Returns:
- rounded complex number
Definition at line 496 of file complex.cpp.
| vector qucs::round | ( | vector | v | ) |
Definition at line 1049 of file vector.cpp.
| vector qucs::rtoy | ( | vector | v, |
| nr_complex_t | zref | ||
| ) |
Definition at line 593 of file vector.cpp.
| nr_complex_t qucs::rtoy | ( | const nr_complex_t | r, |
| nr_complex_t | zref | ||
| ) |
Converts reflexion coefficient to admittance.
- Parameters:
-
[in] r reflexion coefficient [in] zref normalisation impedance
- Returns:
- admittance
Definition at line 608 of file complex.cpp.
| vector qucs::rtoz | ( | vector | v, |
| nr_complex_t | zref | ||
| ) |
Definition at line 586 of file vector.cpp.
| nr_complex_t qucs::rtoz | ( | const nr_complex_t | r, |
| nr_complex_t | zref | ||
| ) |
Converts reflexion coefficient to impedance.
- Parameters:
-
[in] r reflexion coefficient [in] zref normalisation impedance
- Returns:
- impedance
Definition at line 590 of file complex.cpp.
| qucs::vector qucs::runavg | ( | qucs::vector | , |
| const int | |||
| ) |
| vector qucs::runavg | ( | const nr_complex_t | x, |
| const int | n | ||
| ) |
Definition at line 1243 of file vector.cpp.
| vector qucs::runavg | ( | vector | v, |
| const int | n | ||
| ) |
Definition at line 1249 of file vector.cpp.
| nr_type_t qucs::scalar | ( | tvector< nr_type_t > | a, |
| tvector< nr_type_t > | b | ||
| ) |
Definition at line 180 of file tvector.cpp.
| nr_double_t qucs::sech | ( | const nr_double_t | d | ) |
| nr_complex_t qucs::sech | ( | const nr_complex_t | z | ) |
Compute complex hyperbolic secant.
- Parameters:
-
[in] z complex angle
- Returns:
- hyperbolic secant of z
Definition at line 343 of file complex.cpp.
| vector qucs::sech | ( | vector | v | ) |
Definition at line 523 of file vector.cpp.
| vector qucs::sign | ( | vector | v | ) |
Definition at line 284 of file vector.cpp.
| nr_double_t qucs::sign | ( | const nr_double_t | d | ) |
| nr_complex_t qucs::sign | ( | const nr_complex_t | z | ) |
complex sign function
compute
![\[ \mathrm{sign}\;z= \mathrm{sign} (re^{i\theta}) = \begin{cases} 1 & \text{if } z=0 \\ e^{i\theta} & \text{else} \end{cases} \]](form_64.png)
- Parameters:
-
[in] z complex number
- Returns:
- sign of z
- Todo:
- Better implementation z/abs(z) is not really stable
Definition at line 435 of file complex.cpp.
| vector qucs::signum | ( | vector | v | ) |
Definition at line 278 of file vector.cpp.
| nr_double_t qucs::signum | ( | const nr_double_t | d | ) |
| nr_complex_t qucs::signum | ( | const nr_complex_t | z | ) |
complex signum function
compute
![\[ \mathrm{signum}\;z= \mathrm{signum} (re^{i\theta}) = \begin{cases} 0 & \text{if } z=0 \\ e^{i\theta} & \text{else} \end{cases} \]](form_63.png)
- Parameters:
-
[in] z complex number
- Returns:
- signum of z
- Todo:
- Better implementation z/abs(z) is not really stable
Definition at line 416 of file complex.cpp.
| nr_double_t qucs::sin | ( | const nr_double_t | arg | ) |
| nr_complex_t qucs::sin | ( | const nr_complex_t | z | ) |
Compute complex sine.
- Parameters:
-
[in] z complex angle
- Returns:
- sine of z
Definition at line 66 of file complex.cpp.
| vector qucs::sin | ( | vector | v | ) |
Definition at line 457 of file vector.cpp.
| vector qucs::sinc | ( | vector | v | ) |
Definition at line 331 of file vector.cpp.
| nr_double_t qucs::sinc | ( | const nr_double_t | d | ) |
| nr_complex_t qucs::sinc | ( | const nr_complex_t | z | ) |
Cardinal sine.
Compute 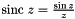
- Parameters:
-
[in] z complex number
- Returns:
- cardianal sine of z
Definition at line 448 of file complex.cpp.
| nr_double_t qucs::sinh | ( | const nr_double_t | arg | ) |
| nr_complex_t qucs::sinh | ( | const nr_complex_t | z | ) |
Compute complex hyperbolic sine.
- Parameters:
-
[in] z complex arc
- Returns:
- hyperbolic sine of z
Definition at line 144 of file complex.cpp.
| vector qucs::sinh | ( | vector | v | ) |
Definition at line 505 of file vector.cpp.
| static int qucs::sortfunc | ( | struct nodelist_t * | n | ) | [static] |
Definition at line 182 of file nodelist.cpp.
| nr_double_t qucs::sqr | ( | const nr_double_t | r | ) |
| matvec qucs::sqr | ( | matvec | a | ) |
Definition at line 489 of file matvec.cpp.
| matrix qucs::sqr | ( | matrix | a | ) |
| nr_complex_t qucs::sqr | ( | const nr_complex_t | z | ) |
Square of complex number.
- Parameters:
-
[in] z complex number
- Returns:
- squared complex number
Definition at line 673 of file complex.cpp.
| vector qucs::sqr | ( | vector | v | ) |
Definition at line 1055 of file vector.cpp.
| nr_double_t qucs::sqrt | ( | const nr_double_t | d | ) |
| nr_complex_t qucs::sqrt | ( | const nr_complex_t | z | ) |
Compute principal value of square root.
Compute the square root of a given complex number (except negative real), and with a branch cut along the negative real axis.
- Parameters:
-
[in] z complex number
- Returns:
- principal value of square root z
Definition at line 271 of file complex.cpp.
| vector qucs::sqrt | ( | vector | v | ) |
Definition at line 380 of file vector.cpp.
| nr_double_t qucs::step | ( | const nr_double_t | d | ) |
Heaviside step function.
The Heaviside step function, H, also called unit step function, is a discontinuous function whose value is zero for negative argument and one for positive argument. For zero by convention, H(0)=0.5
- Parameters:
-
[in] d Heaviside argument
- Returns:
- Heaviside step
- Todo:
- Create Heaviside alias
| nr_complex_t qucs::step | ( | const nr_complex_t | z | ) |
Heaviside step function for complex number.
Apply Heaviside to real and imaginary part
- Parameters:
-
[in] z Heaviside argument
- Returns:
- Heaviside step
- Todo:
Create Heaviside alias
Why not using real heaviside
Definition at line 691 of file complex.cpp.
| vector qucs::step | ( | vector | v | ) |
Definition at line 1061 of file vector.cpp.
| matrix qucs::stoa | ( | matrix | s, |
| nr_complex_t | z1, | ||
| nr_complex_t | z2 | ||
| ) |
Converts chain matrix to scattering parameters.
Converts scattering parameters to chain matrix. Formulae are given by [5] tab 1. and are remembered here:
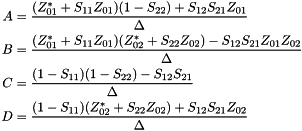
Where:
![\[ \Delta = 2 S_{21}\sqrt{\Re\text{e}\;Z_{01}\Re\text{e}\;Z_{02}} \]](form_97.png)
- Bug:
- Do not need fabs
- Parameters:
-
[in] s Scattering matrix [in] z1 impedance at input 1 [in] z2 impedance at input 2
- Returns:
- Chain matrix
- Note:
- Assert 2 by 2 matrix
- Todo:
- Why not s,z1,z2 const
Definition at line 1181 of file matrix.cpp.
| matrix qucs::stog | ( | matrix | s, |
| nr_complex_t | z1, | ||
| nr_complex_t | z2 | ||
| ) |
Definition at line 1331 of file matrix.cpp.
| matrix qucs::stoh | ( | matrix | s, |
| nr_complex_t | z1, | ||
| nr_complex_t | z2 | ||
| ) |
Converts scattering parameters to hybrid matrix.
Converts chain matrix to scattering parameters Formulae are given by [5] and are remembered here:
Where  is:
is:
![\[ \Delta=(1-S_{11})(Z_{02}^*+S_{22}Z_{02})+S_{12}S_{21}Z_{02} \]](form_102.png)
- Bug:
- {Programmed formulae are valid only for Z real}
- Parameters:
-
[in] s Scattering matrix [in] z1 impedance at input 1 [in] z2 impedance at input 2
- Returns:
- hybrid matrix
- Note:
- Assert 2 by 2 matrix
- Todo:
- Why not s,z1,z2 const
Definition at line 1269 of file matrix.cpp.
| matvec qucs::stos | ( | matvec | , |
| vector | , | ||
| nr_complex_t | z0 = 50.0 |
||
| ) |
| matvec qucs::stos | ( | matvec | , |
| nr_complex_t | , | ||
| vector | |||
| ) |
| matvec qucs::stos | ( | matvec | , |
| vector | , | ||
| vector | |||
| ) |
| matrix qucs::stos | ( | matrix | , |
| vector | , | ||
| nr_complex_t | z0 = 50.0 |
||
| ) |
| matrix qucs::stos | ( | matrix | , |
| nr_complex_t | , | ||
| vector | |||
| ) |
| matrix qucs::stos | ( | matrix | , |
| vector | , | ||
| vector | |||
| ) |
| matvec qucs::stos | ( | matvec | s, |
| qucs::vector | zref, | ||
| qucs::vector | z0 | ||
| ) |
Definition at line 568 of file matvec.cpp.
| matvec qucs::stos | ( | matvec | s, |
| nr_complex_t | zref, | ||
| nr_complex_t | z0 | ||
| ) |
Definition at line 577 of file matvec.cpp.
| matvec qucs::stos | ( | matvec | s, |
| nr_double_t | zref, | ||
| nr_double_t | z0 | ||
| ) |
Definition at line 582 of file matvec.cpp.
| matvec qucs::stos | ( | matvec | s, |
| qucs::vector | zref, | ||
| nr_complex_t | z0 | ||
| ) |
Definition at line 586 of file matvec.cpp.
| matvec qucs::stos | ( | matvec | s, |
| nr_complex_t | zref, | ||
| qucs::vector | z0 | ||
| ) |
Definition at line 590 of file matvec.cpp.
| matrix qucs::stos | ( | matrix | s, |
| qucs::vector | zref, | ||
| qucs::vector | z0 | ||
| ) |
S params to S params.
Convert scattering parameters with the reference impedance 'zref' to scattering parameters with the reference impedance 'z0'.
Detail are given in [1], under equation (32)
New scatering matrix  is:
is:
![\[ S'=A^{-1}(S-\Gamma^+)(I-\Gamma S)^{-1}A^+ \]](form_72.png)
Where x^+ is the adjoint (or complex tranposate) of x, I the identity matrix and  is diagonal the matrix such as:
is diagonal the matrix such as:  and
and  the diagonal matrix such as:
the diagonal matrix such as:
![\[ A_i = \frac{(1-r_i^*)\sqrt{|1-r_ir_i^*|}}{|1-r_i|} \]](form_75.png)
Where  is the complex conjugate of
is the complex conjugate of 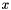 and
and  is wave reflexion coefficient of
is wave reflexion coefficient of  with respect to
with respect to  (where
(where  is the new impedance and
is the new impedance and 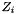 is the old impedance), ie:
is the old impedance), ie:
![\[ r_i = \frac{Z_i'-Z_i}{Z_i'-Z_i^*} \]](form_82.png)
- Parameters:
-
[in] s original S matrix [in] zref original reference impedance [in] z0 new reference impedance
- Bug:
- This formula is valid only for real z!
- Todo:
Correct documentation about standing waves [1-4]
Implement Speciale implementation [2-3] if applicable
- Returns:
- Renormalized scattering matrix
- Todo:
- s, zref and z0 const
Definition at line 890 of file matrix.cpp.
| matrix qucs::stos | ( | matrix | s, |
| nr_complex_t | zref, | ||
| nr_complex_t | z0 | ||
| ) |
S renormalization with all part identic.
- Parameters:
-
[in] s original S matrix [in] zref original reference impedance [in] z0 new reference impedance
- Todo:
- Why not inline
- Returns:
- Renormalized scattering matrix
- Todo:
- s, zref and z0 const
Definition at line 910 of file matrix.cpp.
| matrix qucs::stos | ( | matrix | s, |
| nr_double_t | zref, | ||
| nr_double_t | z0 | ||
| ) |
S renormalization with all part identic and real.
- Parameters:
-
[in] s original S matrix [in] zref original reference impedance [in] z0 new reference impedance
- Todo:
- Why not inline
- Returns:
- Renormalized scattering matrix
- Todo:
- s, zref and z0 const
Definition at line 923 of file matrix.cpp.
| matrix qucs::stos | ( | matrix | s, |
| qucs::vector | zref, | ||
| nr_complex_t | z0 | ||
| ) |
S renormalization (variation)
- Parameters:
-
[in] s original S matrix [in] zref original reference impedance [in] z0 new reference impedance
- Todo:
- Why not inline
- Returns:
- Renormalized scattering matrix
- Todo:
- s, zref and z0 const
Definition at line 935 of file matrix.cpp.
| matrix qucs::stos | ( | matrix | s, |
| nr_complex_t | zref, | ||
| qucs::vector | z0 | ||
| ) |
S renormalization (variation)
- Parameters:
-
[in] s original S matrix [in] zref original reference impedance [in] z0 new reference impedance
- Todo:
Why not inline
s, zref and z0 const
- Returns:
- Renormalized scattering matrix
Definition at line 947 of file matrix.cpp.
| matvec qucs::stoy | ( | matvec | , |
| vector | |||
| ) |
| matrix qucs::stoy | ( | matrix | , |
| vector | |||
| ) |
| matvec qucs::stoy | ( | matvec | s, |
| qucs::vector | z0 | ||
| ) |
Definition at line 595 of file matvec.cpp.
| matvec qucs::stoy | ( | matvec | s, |
| nr_complex_t | z0 | ||
| ) |
Definition at line 602 of file matvec.cpp.
| matrix qucs::stoy | ( | matrix | s, |
| qucs::vector | z0 | ||
| ) |
Scattering parameters to admittance matrix.
Convert scattering parameters to admittance matrix. According to [1] eq (19):
![\[ Z=F^{-1} (I- S)^{-1} (SG + G^+) F \]](form_83.png)
Where  is the scattering matrix,
is the scattering matrix,  is the adjoint of x, I the identity matrix. The matrix F and G are diagonal matrix defined by:
is the adjoint of x, I the identity matrix. The matrix F and G are diagonal matrix defined by:
Using the well know formula 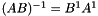 , we derivate:
, we derivate:
![\[ Y=F^{-1} (SG+G^+)^{-1} (I-S) F \]](form_93.png)
- Parameters:
-
[in] s Scattering matrix [in] z0 Normalisation impedance
- Note:
- We could safely drop the
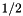 in
in  because we compute
because we compute 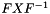 and therefore
and therefore 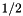 will simplify.
will simplify.
- Bug:
- not correct if zref is complex
- Todo:
- s and z0 const
- Returns:
- Admittance matrix
Definition at line 1082 of file matrix.cpp.
| matrix qucs::stoy | ( | matrix | s, |
| nr_complex_t | z0 | ||
| ) |
Convert scattering pto adminttance parameters identic case.
- Parameters:
-
[in] S Scattering matrix [in] z0 Normalisation impedance
- Returns:
- Admittance matrix
- Todo:
Why not inline
s and z0 const
Definition at line 1101 of file matrix.cpp.
| matvec qucs::stoz | ( | matvec | , |
| vector | |||
| ) |
| matrix qucs::stoz | ( | matrix | , |
| vector | |||
| ) |
| matvec qucs::stoz | ( | matvec | s, |
| qucs::vector | z0 | ||
| ) |
Definition at line 619 of file matvec.cpp.
| matvec qucs::stoz | ( | matvec | s, |
| nr_complex_t | z0 | ||
| ) |
Definition at line 626 of file matvec.cpp.
| matrix qucs::stoz | ( | matrix | s, |
| qucs::vector | z0 | ||
| ) |
Scattering parameters to impedance matrix.
Convert scattering parameters to impedance matrix. According to [1] eq (19):
![\[ Z=F^{-1} (I- S)^{-1} (SG + G^+) F \]](form_83.png)
Where  is the scattering matrix,
is the scattering matrix,  is the adjoint of x, I the identity matrix. The matrix F and G are diagonal matrix defined by:
is the adjoint of x, I the identity matrix. The matrix F and G are diagonal matrix defined by:
- Parameters:
-
[in] s Scattering matrix [in] z0 Normalisation impedance
- Note:
- We could safely drop the
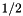 in
in 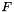 because we compute
because we compute 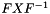 and therefore
and therefore 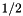 will simplify.
will simplify.
- Bug:
- not correct if zref is complex
- Todo:
- s, z0 const
- Returns:
- Impedance matrix
Definition at line 973 of file matrix.cpp.
| matrix qucs::stoz | ( | matrix | s, |
| nr_complex_t | z0 | ||
| ) |
Scattering parameters to impedance matrix identic case.
- Parameters:
-
[in] s Scattering matrix [in] z0 Normalisation impedance
- Returns:
- Impedance matrix
- Todo:
Why not inline?
s and z0 const?
Definition at line 992 of file matrix.cpp.
| nr_type_t qucs::sum | ( | tvector< nr_type_t > | a | ) |
Definition at line 196 of file tvector.cpp.
| nr_complex_t qucs::sum | ( | vector | v | ) |
Definition at line 260 of file vector.cpp.
| nr_double_t qucs::tan | ( | const nr_double_t | arg | ) |
| nr_complex_t qucs::tan | ( | const nr_complex_t | z | ) |
Compute complex tangent.
- Parameters:
-
[in] z complex angle
- Returns:
- tangent of z
Definition at line 75 of file complex.cpp.
| vector qucs::tan | ( | vector | v | ) |
Definition at line 481 of file vector.cpp.
| nr_double_t qucs::tanh | ( | const nr_double_t | arg | ) |
| nr_complex_t qucs::tanh | ( | const nr_complex_t | z | ) |
Compute complex hyperbolic tangent.
- Parameters:
-
[in] z complex arc
- Returns:
- hyperbolic tangent of z
Definition at line 153 of file complex.cpp.
| vector qucs::tanh | ( | vector | v | ) |
Definition at line 547 of file vector.cpp.
| tmatrix<nr_type_t> qucs::teye | ( | int | n | ) |
Definition at line 247 of file tmatrix.cpp.
| matrix qucs::transpose | ( | matrix | a | ) |
Matrix transposition.
- Parameters:
-
[in] a Matrix to transpose
- Todo:
add transpose in place
a is const
Definition at line 492 of file matrix.cpp.
| matvec qucs::transpose | ( | matvec | a | ) |
Definition at line 560 of file matvec.cpp.
| nr_double_t qucs::trunc | ( | nr_double_t | arg | ) |
| nr_complex_t qucs::trunc | ( | const nr_complex_t | z | ) |
Complex trunc Apply round to integer, towards zero to real and imaginary part.
- Parameters:
-
[in] z complex number
- Returns:
- rounded complex number
Definition at line 512 of file complex.cpp.
| matvec qucs::twoport | ( | matvec | m, |
| char | in, | ||
| char | out | ||
| ) |
Definition at line 661 of file matvec.cpp.
| matrix qucs::twoport | ( | matrix | m, |
| char | in, | ||
| char | out | ||
| ) |
Generic conversion matrix.
This function converts 2x2 matrices from any of the matrix forms Y, Z, H, G and A to any other. Also converts S<->(A, T, H, Y and Z) matrices. Convertion assumed:
Y->Y, Y->Z, Y->H, Y->G, Y->A, Y->S, Z->Y, Z->Z, Z->H, Z->G, Z->A, Z->S, H->Y, H->Z, H->H, H->G, H->A, H->S, G->Y, G->Z, G->H, G->G, G->A, G->S, A->Y, A->Z, A->H, A->G, A->A, A->S, S->Y, S->Z, S->H, S->G, S->A, S->S, S->T,T->T,T->S
- Note:
- assert 2x2 matrix
- Parameters:
-
[in] m base matrix [in] in matrix [in] out matrix
- Returns:
- matrix given by format out
- Todo:
- m, in, out const
Definition at line 1594 of file matrix.cpp.
| vector qucs::unwrap | ( | vector | v, |
| nr_double_t | tol, | ||
| nr_double_t | step | ||
| ) |
Definition at line 244 of file vector.cpp.
| vector qucs::w2dbm | ( | vector | v | ) |
Definition at line 1207 of file vector.cpp.
| nr_double_t qucs::xhypot | ( | const nr_double_t | a, |
| const nr_double_t | b | ||
| ) |
Euclidean distance function.
The xhypot() function returns 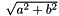 . This is the length of the hypotenuse of a right-angle triangle with sides of length a and b, or the distance of the point (a,b) from the origin.
. This is the length of the hypotenuse of a right-angle triangle with sides of length a and b, or the distance of the point (a,b) from the origin.
- Parameters:
-
[in] a first length [in] b second length
- Returns:
- Euclidean distance from (0,0) to (a,b):
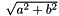
| vector qucs::xhypot | ( | vector | v, |
| const nr_complex_t | z | ||
| ) |
Definition at line 290 of file vector.cpp.
| vector qucs::xhypot | ( | vector | v, |
| const nr_double_t | d | ||
| ) |
Definition at line 296 of file vector.cpp.
| vector qucs::xhypot | ( | const nr_complex_t | z, |
| vector | v | ||
| ) |
Definition at line 302 of file vector.cpp.
| vector qucs::xhypot | ( | const nr_double_t | d, |
| vector | v | ||
| ) |
Definition at line 308 of file vector.cpp.
| vector qucs::xhypot | ( | vector | v1, |
| vector | v2 | ||
| ) |
Definition at line 314 of file vector.cpp.
| nr_double_t qucs::xhypot | ( | const nr_complex_t | a, |
| const nr_complex_t | b | ||
| ) |
Euclidean distance function for complex argument.
The xhypot() function returns 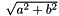 . This is the length of the hypotenuse of a right-angle triangle with sides of length a and b, or the distance of the point (a,b) from the origin.
. This is the length of the hypotenuse of a right-angle triangle with sides of length a and b, or the distance of the point (a,b) from the origin.
- Parameters:
-
[in] a first length [in] b second length
- Returns:
- Euclidean distance from (0,0) to (a,b):
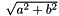
Definition at line 465 of file complex.cpp.
| nr_double_t qucs::xhypot | ( | nr_double_t | a, |
| nr_complex_t | b | ||
| ) |
Euclidean distance function for a double b complex.
Definition at line 478 of file complex.cpp.
| nr_double_t qucs::xhypot | ( | nr_complex_t | a, |
| nr_double_t | b | ||
| ) |
Euclidean distance function for b double a complex.
Definition at line 484 of file complex.cpp.
| nr_complex_t qucs::yn | ( | const int | n, |
| const nr_complex_t | z | ||
| ) |
Bessel function of second kind.
- Parameters:
-
[in] n order [in] z argument
- Returns:
- Bessel function of second kind of order n
- Bug:
- Not implemented
Definition at line 742 of file complex.cpp.
Definition at line 1141 of file vector.cpp.
| vector qucs::ytor | ( | vector | v, |
| nr_complex_t | zref | ||
| ) |
Definition at line 579 of file vector.cpp.
| nr_complex_t qucs::ytor | ( | const nr_complex_t | y, |
| nr_complex_t | zref | ||
| ) |
Converts admittance to reflexion coefficient.
- Parameters:
-
[in] y admitance [in] zref normalisation impedance
- Returns:
- reflexion coefficient
Definition at line 599 of file complex.cpp.
| matvec qucs::ytos | ( | matvec | , |
| vector | |||
| ) |
| matrix qucs::ytos | ( | matrix | , |
| vector | |||
| ) |
| matvec qucs::ytos | ( | matvec | y, |
| qucs::vector | z0 | ||
| ) |
Definition at line 607 of file matvec.cpp.
| matvec qucs::ytos | ( | matvec | y, |
| nr_complex_t | z0 | ||
| ) |
Definition at line 614 of file matvec.cpp.
| matrix qucs::ytos | ( | matrix | y, |
| qucs::vector | z0 | ||
| ) |
Admittance matrix to scattering parameters.
Convert admittance matrix to scattering parameters. Using the same methodology as [1] eq (16-19), but writing (16) as  , ie
, ie
![\[ S=F(I-G^+Y)(I-GY)^{-1}F^{-1} \]](form_95.png)
Where 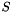 is the scattering matrix,
is the scattering matrix,  is the adjoint of x, I the identity matrix. The matrix F and G are diagonal matrix defined by:
is the adjoint of x, I the identity matrix. The matrix F and G are diagonal matrix defined by:
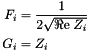
Using the well know formula 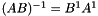 , we derivate:
, we derivate:
![\[ Y=F^{-1} (SG+G^+)^{-1} (I-S) F \]](form_93.png)
- Parameters:
-
[in] y admittance matrix [in] z0 Normalisation impedance
- Note:
- We could safely drop the
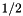 in
in 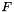 because we compute
because we compute 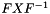 and therefore
and therefore 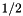 will simplify.
will simplify.
- Bug:
- not correct if zref is complex
- Todo:
- why not y and z0 const
- Returns:
- Scattering matrix
Definition at line 1133 of file matrix.cpp.
| matrix qucs::ytos | ( | matrix | y, |
| nr_complex_t | z0 | ||
| ) |
Convert Admittance matrix to scattering parameters identic case.
- Parameters:
-
[in] y Admittance matrix [in] z0 Normalisation impedance
- Returns:
- Scattering matrix
- Todo:
Why not inline
y and z0 const
Definition at line 1151 of file matrix.cpp.
| matvec qucs::ytoz | ( | matvec | y | ) |
Definition at line 651 of file matvec.cpp.
| matrix qucs::ytoz | ( | matrix | y | ) |
Convert admittance matrix to impedance matrix.
Convert  matrix to
matrix to 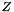 matrix using well known relation
matrix using well known relation 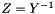
- Parameters:
-
[in] y admittance matrix
- Returns:
- Impedance matrix
- Note:
- Check if y matrix is a square matrix
Definition at line 1380 of file matrix.cpp.
| vector qucs::ztor | ( | vector | v, |
| nr_complex_t | zref | ||
| ) |
Definition at line 572 of file vector.cpp.
| nr_complex_t qucs::ztor | ( | const nr_complex_t | z, |
| nr_complex_t | zref | ||
| ) |
Converts impedance to reflexion coefficient.
- Parameters:
-
[in] z impedance [in] zref normalisation impedance
- Returns:
- reflexion coefficient
Definition at line 581 of file complex.cpp.
| matvec qucs::ztos | ( | matvec | , |
| vector | |||
| ) |
| matrix qucs::ztos | ( | matrix | , |
| vector | |||
| ) |
| matvec qucs::ztos | ( | matvec | z, |
| qucs::vector | z0 | ||
| ) |
Definition at line 631 of file matvec.cpp.
| matvec qucs::ztos | ( | matvec | z, |
| nr_complex_t | z0 | ||
| ) |
Definition at line 638 of file matvec.cpp.
| matrix qucs::ztos | ( | matrix | z, |
| qucs::vector | z0 | ||
| ) |
Convert impedance matrix scattering parameters.
Convert scattering parameters to impedance matrix. According to [1] eq (18):
![\[ S=F(Z-G^+)(Z+G)^{-1} F^{-1} \]](form_89.png)
Where 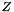 is the scattering matrix,
is the scattering matrix,  is the adjoint of x, I the identity matrix. The matrix F and G are diagonal matrix defined by:
is the adjoint of x, I the identity matrix. The matrix F and G are diagonal matrix defined by:
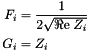
- Parameters:
-
[in] Z Impedance matrix [in] z0 Normalisation impedance
- Returns:
- Scattering matrix
- Note:
- We could safely drop the
 in
in  because we compute
because we compute  and therefore
and therefore 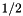 will simplify.
will simplify.
- Bug:
- not correct if zref is complex
- Todo:
- z and z0 const?
Definition at line 1018 of file matrix.cpp.
| matrix qucs::ztos | ( | matrix | z, |
| nr_complex_t | z0 | ||
| ) |
Convert impedance matrix to scattering parameters identic case.
- Parameters:
-
[in] Z Impedance matrix [in] z0 Normalisation impedance
- Returns:
- Scattering matrix
- Todo:
Why not inline
z and z0 const
Definition at line 1037 of file matrix.cpp.
| matvec qucs::ztoy | ( | matvec | z | ) |
Definition at line 643 of file matvec.cpp.
| matrix qucs::ztoy | ( | matrix | z | ) |
impedance matrix to admittance matrix.
Convert impedance matrix to admittance matrix. By definition 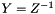
- Parameters:
-
[in] z impedance matrix
- Returns:
- Admittance matrix
- Todo:
Why not inline
z const
Definition at line 1050 of file matrix.cpp.
![\[ \operatorname{fix} d=\begin{cases} \operatorname{floor} d & \text{if } d > 0 \\ \operatorname{ceil} d & \text{else} \end{cases} \]](form_122.png)
![\[ \begin{cases} \exp r & \text{if } r < \text{M\_LIMEXP} \\ \exp (\text{M\_LIMEXP})\left[1.0 + (r - \text{M\_LIMEXP})\right] & \text{else} \end{cases} \]](form_118.png)
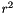
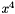
![\[ \mathrm{sign}\;d= = \begin{cases} 1 & \text{if } d\ge 0 \\ -1 & \text{if } d<0 \end{cases} \]](form_120.png)
![\[ \mathrm{signum}\;d= = \begin{cases} O & \text{if } d=0 \\ 1 & \text{if } d>0 \\ -1 & \text{if } d<0 \end{cases} \]](form_119.png)

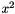
 1.7.6.1
1.7.6.1